函数极限与连续复盘

函数的概念与特性

算原函数:
若给 $f(x)+xf(?x)=x$, 应学会写 $f(?x)?xf(x)=?x$, 消去 $f(?x)$, 得 $f(x)=\frac{x+x^2}{1+x^2}$.

题目:
单f(x)核心在于凑!
例 1.1 设 $f\left(x+\frac{1}{x}\right)=\frac{x+x^3}{1+x^4},x?2$, 则 $f(x)=$
多f(x)核心在于创建方程!
例 1.2 设函数 $f(x)$ 的定义域为 $(0,+\infty )$, 且满足 $2f(x)+x^{2}f\left(\frac{1}{x}\right)=\frac{x^2+2x}{\sqrt{1+x^2}}$, 则 $f(x)=$

反函数

第一:严格单调函数必有反函数

第二, 若把 $x=f^{?1}(y)$ 与 $y=f(x)$ 的图形画在同一坐标系中, 则它们完全重合. 只有把 $y=f(x)$ 的反函数 $x=f^{?1}(y)$ 写成 $y=f^{?1}(x)$ 后, 它们的图形才关于 $y=x$ 对称, 事实上这也是字母 $x$ 与 $y$ 互换的结果.
题目:
求反函数(就是法则互换)
例 1.3 求函数 $y=f(x)=\ln \left(x+\sqrt{x^2+1}\right)$ 的反函数 $f^{?1}(x)$ 的表达式及其定义域.

复合函数

都先要把内函数求出来,根据内函数的变化去完成复合函数的要求
题目:
单复合
例 1.4 设 $f(x)=x^2,f[\phi (x)]=?x^2+2x+3$, 且 $\phi (x)?0$, 求 $\phi (x)$ 及其定义域与值域.
分段复合
例 1.5 设 $g(x)=\{\begin{array}{l}2?x,x?0,\\ 2+x,x>0,\end{array}f(x)=\{\begin{array}{ll}{x}^2,& x<0,\\ ?x?1,& x?0,\end{array}$ 则 $g[f(x)]=$

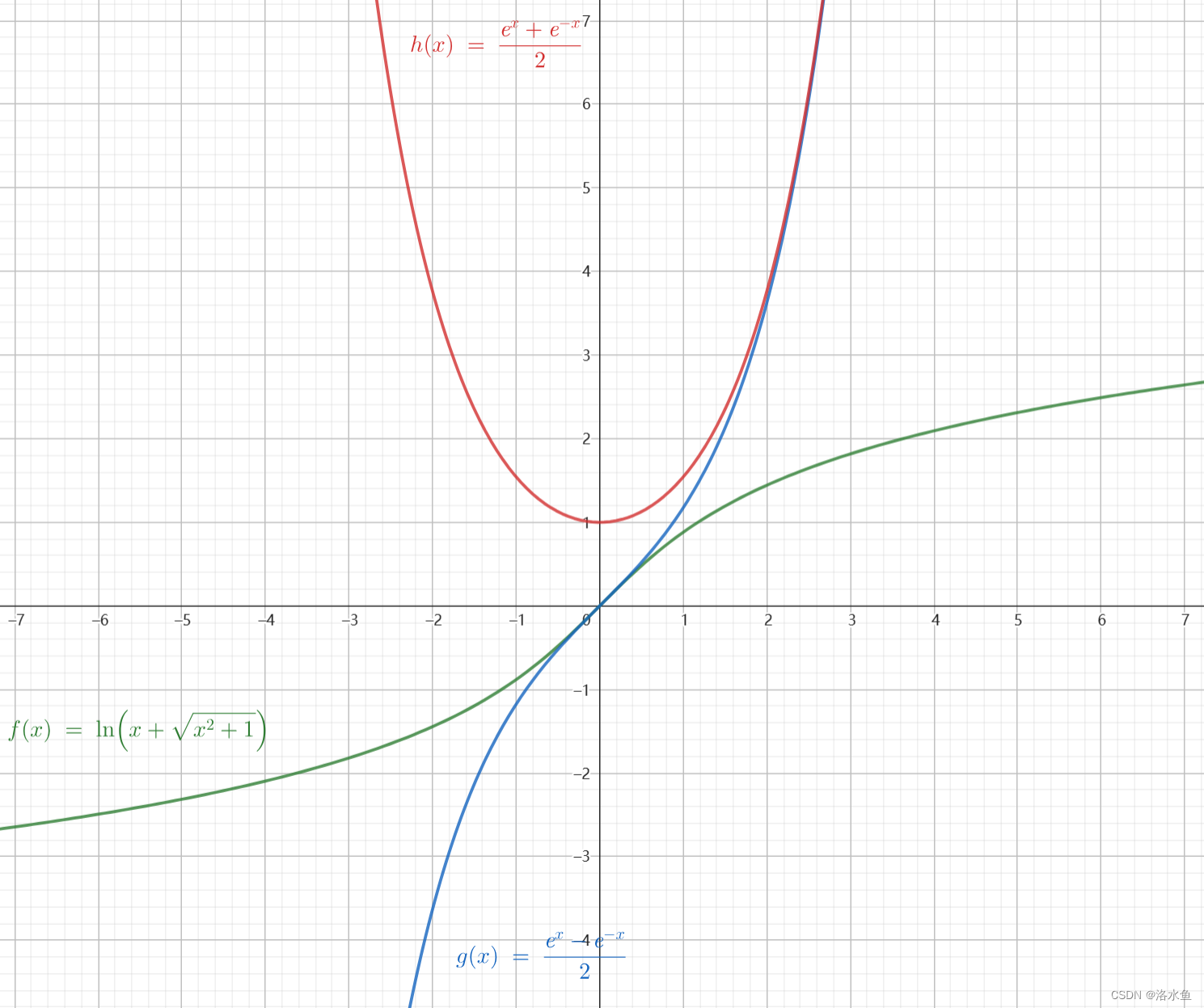
重要函数图像

【注】(1)
函数 $y=\ln \left(x+\sqrt{x^2+1}\right)$ 叫作反双曲正弦函数,
函数 $y=\frac{{\mathrm{e}}^x?{\mathrm{e}}^{?x}}{2}$叫作双曲正弦函数,
考生应记住这两个函数的图像.
(2) $y=\frac{{\mathrm{e}}^x+{\mathrm{e}}^{?x}}{2}$ 叫作双曲余弦函数, 它是偶函数, 是一种特殊的悬链线.
如图所示:

三个重要结论

(1) $x\to 0$ 时, $\ln \left(x+\sqrt{x^2+1}\right)～x$

(2) ${\left[\ln \left(x+\sqrt{x^2+1}\right)\right]}^{\prime }=\frac{1}{\sqrt{x^2+1}}$,
于是 $\int \frac{1}{\sqrt{x^2+1}}\mathrm{d}x=\ln \left(x+\sqrt{x^2+1}\right)+C$.

(3) 由于 $y=\ln \left(x+\sqrt{x^2+1}\right)$ 是奇函数, 于是 ${\int }_{?1}^1\left[\ln \left(x+\sqrt{x^2+1}\right)+x^2\right]\mathrm{d}x={\int }_{?1}^1{x}^2\mathrm{d}x=\frac{2}{3}$.

隐函数

如 $x+y^3?1=0$ 就表示一个隐函数, 且可显化为 $y=\sqrt[3]{1?x}$; 再如 $\sin (xy)=\ln \frac{x+\mathrm{e}}{y}+1$ 也表示一个隐函数, 但不易显化.

函数的四种特性

有界性

设 $f(x)$ 的定义域为 $D$, 数集 $I?D$. 如果存在某个正数 $M$, 使对任一 $x\in I$, 有 $|f(x)|?M$, 则称 $f(x)$ 在 $I$ 上有界; 如果这样的 $M$ 不存在, 则称 $f(x)$ 在 $I$ 上无界.

(1) 从几何上看, 如果在给定的区间, 函数 $y=f(x)$ 的图形能够被直线 $y=?M$ 和 $y=M$ “完全包起来”, 则为有界; 从解析上说，如果找到某个正数 $M$ ，使得 $|f(x)|?M$ ，则为有界.

(2) 有界还是无界的讨论首先需指明区间 $I$, 不知区间, 无法谈论有界性. 比如 $y=\frac{1}{x}$ 在 $(2,+\infty )$内有界, 但在 $(0,2)$ 内无界.

(3) 事实上, 只要在区间 $I$ 上或其端点处存在点 $x_0$, 使得 ${\lim }_{x\to x_0}f(x)$ 的值为无穷大, 则没有任何两条直线 $y=?M$ 和 $y=M$ 可以把 $I$ 上的 $f(x)$ “包起来”, 这就叫无界.
题目:
例 1.6 证明函数 $f(x)=\frac{x}{1+x^2}$ 在 $(?\infty ,+\infty )$ 内有界.(其实就是证有: $|f(x)|?M$)
其实就是看绝对值f(x)有没有最大值,可以利用不等式(因为可以化为单因素影响)
证明 当 $x\ne 0$ 时,

$$|f(x)|=\frac{|x|}{1+x^2}=\frac{1}{\frac{1}{|x|}+|x|}$$

由不等式 $\frac{a+b}{2}?\sqrt{ab}(a,b>0)$, 有 $\frac{1}{|x|}+|x|?2\sqrt{\frac{1}{|x|}|x|}=2$, 即 $|f(x)|?\frac{1}{2}$.

当 $x=0$ 时, $f(0)=0$. 综上, 函数 $f(x)$ 在 $(?\infty ,+\infty )$ 内有界.

单调性

对任意 $x_1,x_2\in D,x_1\ne x_2$, 有

$$\begin{array}{rl}& f(x)\text{?是单调增函数?}?\left(x_1?x_2\right)\left[f\left(x_1\right)?f\left(x_2\right)\right]>0;\\ & f(x)\text{?是单调减函数?}?\left(x_1?x_2\right)\left[f\left(x_1\right)?f\left(x_2\right)\right]<0;\\ & f(x)\text{?是单调不减函数?}?\left(x_1?x_2\right)\left[f\left(x_1\right)?f\left(x_2\right)\right]?0；\\ & f(x)\text{?是单调不增函数?}?\left(x_1?x_2\right)\left[f\left(x_1\right)?f\left(x_2\right)\right]?0.\end{array}$$
题目
例 1.7 设 $f(x)$ 在 $(?\infty ,+\infty )$ 上有定义, 任给 $x_1,x_2,x_1\ne x_2$, 均有 $\left(x_1?x_2\right)?\left[f\left(x_1\right)?f\left(x_2\right)\right]>0$,则以下函数一定单调增加的是 $()$.
(A) $|f(x)|$
(B) $f(|x|)$
(C) $f(?x)$
(D) $?f(?x)$

例 1.8 设对任意 $x,y$, 都有 $f(x+y)=f(x)+f(y)$, 证明: $f(x)$ 是奇函数.

奇偶性

定义

设 $f(x)$ 的定义域 $D$ 关于原点对称 (若 $x\in D$, 则 $?x\in D$ ).
如果对于任一 $x\in D$, 恒有 $f(?x)=f(x)$,则称 $f(x)$ 为偶函数.
如果对于任 $?x\in D$, 恒有 $f(?x)=?f(x)$, 则称 $f(x)$ 为奇函数.
我们熟知的是, 偶函数的图形关于 $y$ 轴对称, 奇函数的图形关于原点对称.

判断式

【注】
(1) 前提: 定义域关于原点对称.
$f(x)+f(?x)$ 必是偶函数.
如:$\frac{{\mathrm{e}}^x+{\mathrm{e}}^{?x}}{2}$

$f(x)?f(?x)$ 必是奇函数.
如:$\frac{{\mathrm{e}}^x?{\mathrm{e}}^{?x}}{2}$

任何一个函数都可以写成一个奇函数与一个偶函数之和的形式.
$$f(x)=\frac{1}{2}[f(x)+f(?x)]+\frac{1}{2}[f(x)?f(?x)]=u(x)+v(x)$$

复合函数的奇偶性:

$f[\phi (x)]$ (内偶则偶, 内奇同外).

两个要记住的函数奇偶性:

函数 $y=\ln \left(x+\sqrt{x^2+1}\right)$

函数 $y=\frac{{\mathrm{e}}^x?{\mathrm{e}}^{?x}}{2}$

都是奇函数,记住图像

导数的奇偶性性质:

$f(x)$ 奇 $?f^{\prime }(x)$ 偶 $?f^{\prime \prime }(x)$ 奇 $??$.

一种特殊的形式

设对任意的 $x,y$, 都有 $f(x+y)=f(x)+f(y)$, 则 $f(x)$ 是奇函数
证明 令 $x=y=0$, 则 $f(0)=f(0)+f(0)$, 于是 $f(0)=0$, 再令 $y=?x$, 则 $f(0)=f(x)+f(?x)$, 即 $f(?x)=?f(x)$, 故 $f(x)$ 是奇函数.

周期性

设 $f(x)$ 的定义域为 $D$, 如果存在一个正数 $T$, 使得对于任一 $x\in D$, 有 $x\pm T\in D$, 且 $f(x+T)=$ $f(x)$, 则称 $f(x)$ 为周期函数, $T$ 称为 $f(x)$ 的周期.

重要结论

(1)若 $f(x)$ 以 $T$ 为周期，则 $f(ax+b)$ 以 $\frac{T}{|a|}$ 为周期.

(2)若 $g(x)$ 是周期函数, 则复合函数 $f[g(x)]$ 也是周期函数，如 ${\mathrm{e}}^{\sin x},{\cos }^{2}x$ 等.

(3)若 $f(x)$ 是以 $T$ 为周期的可导函数，则 $f^{\prime }(x)$ 也以 $T$ 为周期.

(4)若 $f(x)$ 是以 $T$ 为周期的连续函数, 则只有在 ${\int }_0^{T}f(x)\mathrm{d}x=0$ 时, ${\int }_0^{x}f(t)\mathrm{d}t$ 也以 $T$ 为周期.(不会)

题目:
例 1.9 设函数 $f(x)$ 在 $(?\infty ,+\infty )$ 上满足 $f(x)=f(x?\pi )+\sin x$. 证明: $f(x)$ 是以 $T=2\pi$ 为周期的周期函数.

函数的图像

基本初等函数与初等函数

基本初等函数：常数函数、幂函数、指数函数、对数函数、三角函数、反三角函数.(反对幂指三)
常数函数:

幂函数:

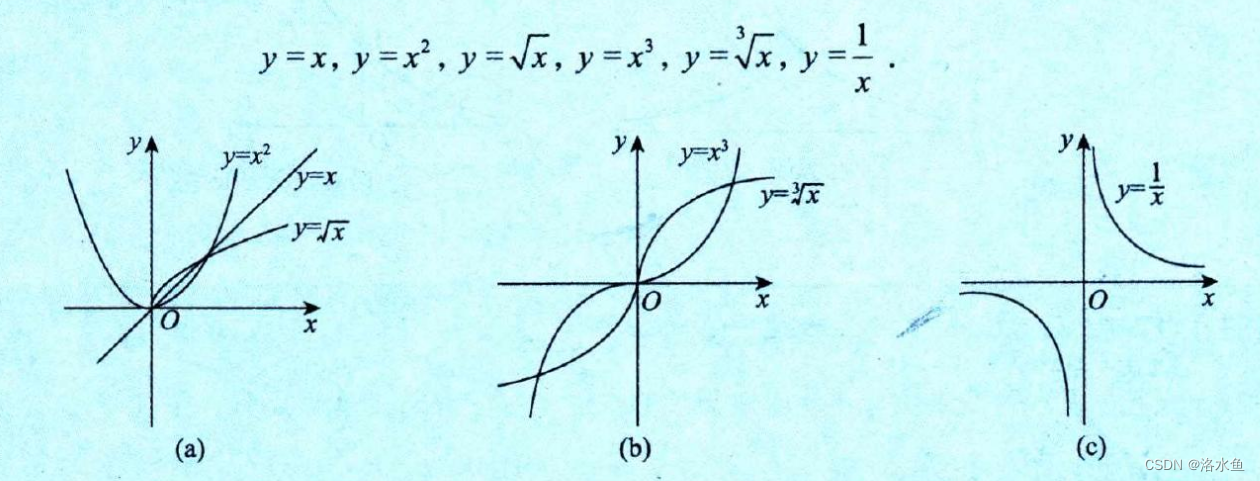
有趣的特性:

当 $x>0$ 时, 由 $y=x$ 与 $y=\sqrt{x},y=\sqrt[3]{x},y=\ln x$ 见图 具有相同的单调性且与 $y=\frac{1}{x}$ 具有相反的单调性,
(1)见到 $\sqrt{u},\sqrt[3]{u}$ 时，可用 $u$ 来研究最值;

(2)见到 $|u|$ 时，由 $|u|=\sqrt{u^2}$ ，可用 $u^2$ 来研究最值;

(3) 见到 $u_1{u}_2{u}_3$ 时, 可用 $\ln \left(u_1{u}_2{u}_3\right)=\ln u_1+\ln u_2+\ln u_3$ 来研究最值;

(4)见到 $\frac{1}{u}$ 时, 可用 $u$ 来研究最值 (结论相反, 即 $\frac{1}{u}$ 与 $u$ 的最大值点、最小值点相反).

指数函数:

指数函数:

函数极限的概念与性质

邻域:
U ( x 0 , δ ) = { x ∣ x 0 ? δ < x < x 0 + δ } = { x ∣ ∣ x ? x 0 ∣ < δ } , U\left(x_{0}, \delta\right)=\left\{x \mid x_{0}-\delta<x<x_{0}+\delta\right\}=\left\{x|| x-x_{0} \mid<\delta\right\}, U(x0?,δ)={x∣x0??δ<x<x0?+δ}={x∣∣x?x0?∣<δ},
左右邻域:
就是去掉绝对值
. { x ∣ 0 < x ? x 0 < δ } \left\{x \mid 0<x-x_{0}<\delta\right\} {x∣0<x?x0?<δ} 称为点 $x_0$ 的右 $\delta$ 邻域, 记作 $U^{+}\left(x_0,\delta \right)$; { x ∣ 0 < x 0 ? x < δ } \left\{x \mid 0<x_{0}-x<\delta\right\} {x∣0<x0??x<δ} 称为点 $x_0$ 的左 $\delta$ 邻域, 记作 $U^{?}\left(x_0,\delta \right)$.

函数极限的定义

这两个图要记,记住两把尺子就好

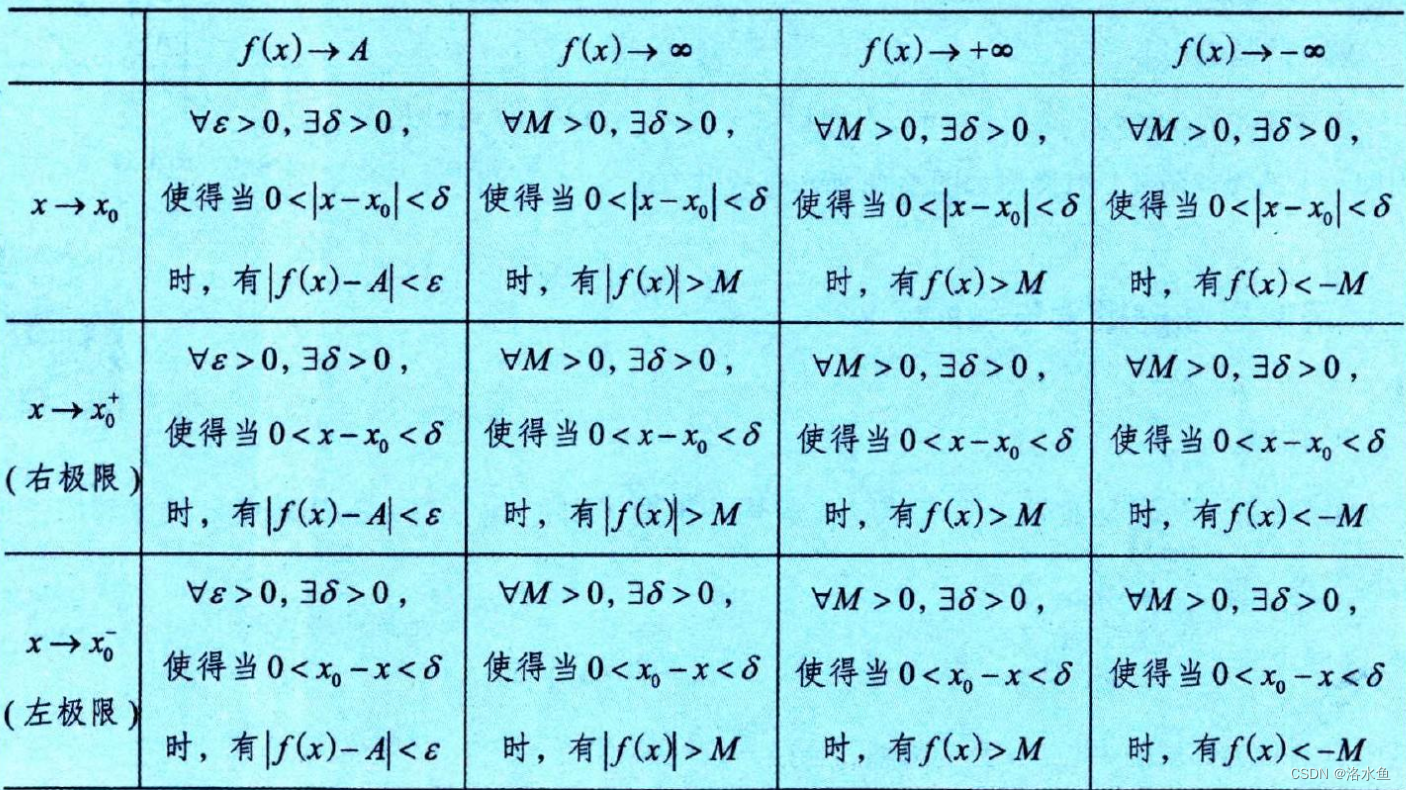
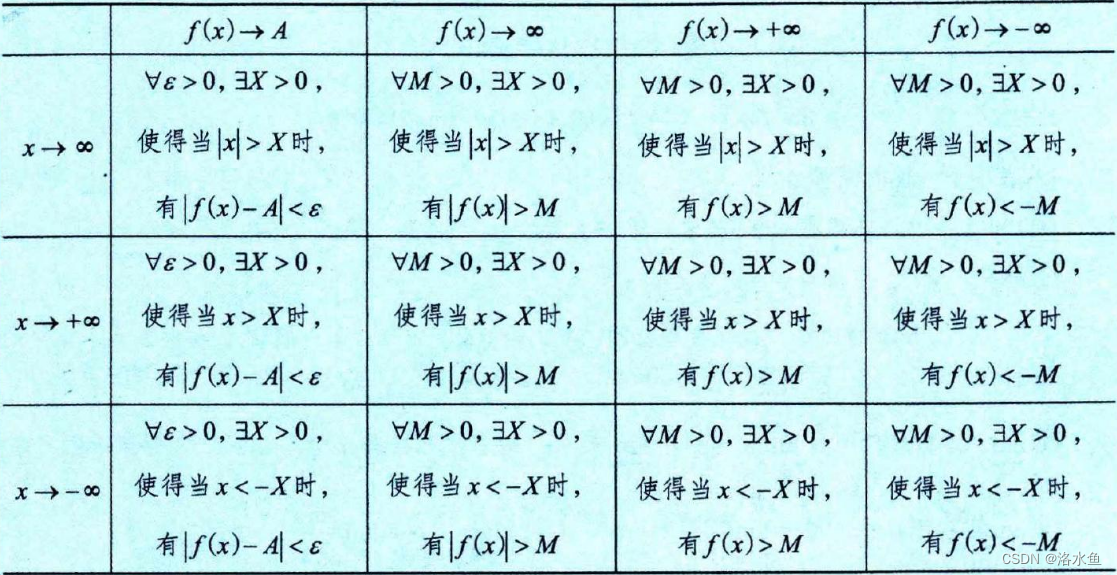
函数极限的性质

(1) 唯一性 .

如果极限 ${\lim }_{x\to x_0}f(x)$ 存在, 那么极限唯一.
函数极限存在的充要条件.
$$\begin{array}{rl}& \underset{x\to x_0}{\lim }f(x)=A?\underset{x\to x_0^{?}}{\lim }f(x)=A\text{?且?}\underset{x\to x_0^{+}}{\lim }f(x)=A,\\ & \underset{x\to x_0}{\lim }f(x)=A?f(x)=A+\alpha (x),\underset{x\to x_0}{\lim }\alpha (x)=0.\end{array}$$
什么情况下要注意左右极限:

(1) ${\lim }_{x\to \infty }{\mathrm{e}}^x$ 不存在, 因为 ${\lim }_{x\to +\infty }{\mathrm{e}}^x=+\infty ,{\lim }_{x\to ?\infty }{\mathrm{e}}^x=0$, 根据 “极限若存在, 必唯一”, 得原极限不存在;

(2) ${\lim }_{x\to 0}\frac{\sin x}{|x|}$ 不存在, 因为 ${\lim }_{x\to 0^{+}}\frac{\sin x}{|x|}={\lim }_{x\to 0^{+}}\frac{\sin x}{x}=1,{\lim }_{x\to 0^{?}}\frac{\sin x}{|x|}={\lim }_{x\to 0^{?}}\frac{\sin x}{?x}=?1$ ；

(3) ${\lim }_{x\to \infty }\arctan x$ 不存在, 因为 ${\lim }_{x\to +\infty }\arctan x=\frac{\pi }{2},{\lim }_{x\to ?\infty }\arctan x=?\frac{\pi }{2}$;

(4) ${\lim }_{x\to 0}[x]$ 不存在, 因为 ${\lim }_{x\to 0^{+}}[x]=0,{\lim }_{x\to 0^{?}}[x]=?1$;

(5)分段函数分段点两侧表达式不同，需分别求左、右极限.

题:
例 1.15 当 $x\to 1$ 时, 函数 $\frac{{\mathrm{e}}^{\frac{1}{x?1}}\ln |1+x|}{\left({\mathrm{e}}^x?1\right)(x?2)}$ 的极限 $()$.
(A) 等于 1
(B) 等于 0
(C) 为 $\infty$
(D) 不存在且不为 $\infty$

(2) 局部有界性 .

如果 ${\lim }_{x\to x_0}f(x)=A$, 则存在正常数 $M$ 和 $\delta$, 使得当 $0<\left|x?x_0\right|<\delta$ 时, 有 $|f(x)|?M$.
(1)注意的是, 极限存在只是函数局部有界的充分条件, 并非必要条件;
(2)若 $y^{\prime }=f(x)$ 在 $[a,b]$ 上为连续函数, 则 $f(x)$ 在 $[a,b]$ 上必定有界;

(3)若 $f(x)$ 在 $(a,b)$ 内为连续函数, 且 ${\lim }_{x\to a^{+}}f(x)$ 与 ${\lim }_{x\to b^{?}}f(x)$ 都存在, 则 $f(x)$ 在 $(a,b)$ 内必定有界;
(4)有界函数与有界函数的和、差、积仍为有界函数.

(3) 局部保号性 .

如果 $f(x)\to A\left(x\to x_0\right)$ 且 $A>0$ (或 $A<0$ ), 那么存在常数 $\delta >0$,使得当 $0<\left|x?x_0\right|<\delta$ 时, 有 $f(x)>0$ (或 $f(x)<0$ ). 如果在 $x_0$ 的某去心邻域内 $f(x)?0($ 或 $f(x)?0)$ 且 ${\lim }_{x\to x_0}f(x)=A$, 则 $A?0($ 或 $A?0)$.

无穷小的定义

无穷小的性质

(1)有限个无穷小的和是无穷小.

(2)有界函数与无穷小的乘积是无穷小.

(3)有限个无穷小的乘积是无穷小 .

常用的等价无穷小

当 $x\to 0$ 时, 常用的等价无穷小有

$$\begin{array}{c}\sin x～x,\tan x～x,\arcsin x～x,\arctan x～x,\ln (1+x)～x,{\mathrm{e}}^x?1～x,\\ a^x?1～x\ln a,1?\cos x～\frac{1}{2}{x}^2,(1+x{)}^a?1～ax.\end{array}$$

无穷大的定义

如果当 $x\to x_0$ (或 $x\to \infty )$ 时, 函数 $|f(x)|$ 无限增大, 那么称函数 $f(x)$ 为当 $x\to x_0($ 或 $x\to \infty )$ 时的无穷大, 记为:
$$\underset{x\to x_0}{\lim }f(x)=\infty \left(\text{?或?}\underset{x\to \infty }{\lim }f(x)=\infty \right).$$

题目:(学会抓大头)
$1.7?1$ 解 ${\lim }_{x\to 0^{+}}\frac{1?{\mathrm{e}}^{\frac{1}{x}}}{x+{\mathrm{e}}^{\frac{1}{x}}}={\lim }_{x\to 0^{+}}\frac{?{\mathrm{e}}^{\frac{1}{x}}}{{\mathrm{e}}^{\frac{1}{x}}}=?1$.

$1.8{\mathrm{e}}^3$ 解 ${\lim }_{x\to \infty }{\left(\frac{x+2}{x?1}\right)}^x={\lim }_{x\to \infty }{\left(\frac{1+\frac{2}{x}}{1?\frac{1}{x}}\right)}^x=\frac{{\lim }_{x\to \infty }{\left(1+\frac{2}{x}\right)}^x}{{\lim }_{x\to \infty }{\left(1?\frac{1}{x}\right)}^x}=\frac{{\mathrm{e}}^2}{{\mathrm{e}}^{?1}}={\mathrm{e}}^3$.

1.16 ${\lim }_{x\to 0^{?}}\frac{\ln \left(1+{\mathrm{e}}^{\frac{2}{x}}\right)}{\ln \left(1+{\mathrm{e}}^{\frac{1}{x}}\right)}=\frac{u=\frac{1}{x}}{{\lim }_{u\to ?\infty }}\frac{\frac{2{\mathrm{e}}^{2u}}{1+{\mathrm{e}}^{2u}}}{\frac{{\mathrm{e}}^u}{1+{\mathrm{e}}^u}}=0,{\lim }_{x\to 0^{?}}a[x]=?a$

计算

【注】
(1) 若 $\lim f(x)$ 存在, $\lim g(x)$ 不存在, 则 $\lim [f(x)\pm g(x)]$ 必不存在. $\to =\lim f(x)\pm \lim g(x)$
(2) 若 $\lim f(x)$ 不存在, $\lim g(x)$ 也不存在, 则 $\lim [f(x)\pm g(x)]$ 不一定不存在.

(3) 若 $\lim f(x)=A\ne 0$, 则 $\lim f(x)g(x)=A\lim g(x)$, 即乘除法中非零因子可往外先提出去.
题目:
1.1 设 $f(x)=u(x)+v(x),g(x)=u(x)?v(x)$, 并设 ${\lim }_{x\to x_0}u(x)$ 与 ${\lim }_{x\to x_0}v(x)$ 都不存在, 下列结论正确的是 ( ).
(A) 若 ${\lim }_{x\to x_0}f(x)$ 不存在, 则 ${\lim }_{x\to x_0}g(x)$ 必存在
(B) 若 ${\lim }_{x\to x_0}f(x)$ 不存在, 则 ${\lim }_{x\to x_0}g(x)$ 必不存在
(C) 若 ${\lim }_{x\to x_0}f(x)$ 存在, 则 ${\lim }_{x\to x_0}g(x)$ 必不存在
(D) 若 ${\lim }_{x\to x_0}f(x)$ 存在, 则 ${\lim }_{x\to x_0}g(x)$ 必存在

重要的隐藏条件

例 1.20 证明:
(1) 若 $\lim \frac{f(x)}{g(x)}=A$, 且 $\lim g(x)=0$, 则 $\lim f(x)=0$ ；

(2) 若 $\lim \frac{f(x)}{g(x)}=A\ne 0$, 且 $\lim f(x)=0$, 则 $\lim g(x)=0$.

证明

(1) 由于 $f(x)=\frac{f(x)}{g(x)}?g(x)$, 则
$$\lim f(x)=\lim \frac{f(x)}{g(x)}?g(x)=\lim \frac{f(x)}{g(x)}?\lim g(x)=A?0=0$$

(2) 由于 $g(x)=\frac{f(x)}{\frac{f(x)}{g(x)}}$, 则 $\lim g(x)=\lim \frac{f(x)}{\frac{f(x)}{g(x)}}=\frac{\lim f(x)}{\lim \frac{f(x)}{g(x)}}=\frac{0}{A}=0$.

洛必达法则

【注 】
(1)一般来说, 洛必达法则是用来计算 “ $\frac{0}{0}$ ” 型或者 “ $\frac{\infty }{\infty }$ ” 型未定式的, 不是 “ $\frac{0}{0}$ ” 型和 “ $\frac{\infty }{\infty }$ ”型, 就不能用洛必达法则.
(2) 如果极限 ${\lim }_{x\to a}\frac{f^{\prime }(x)}{F^{\prime }(x)}$ 仍属于 “ $\frac{0}{0}$ ” 型或者 “ $\frac{\infty }{\infty }$ ” 型,且 $f^{\prime }(x),F^{\prime }(x)$ 继续满足洛必达法则的条件,则可以继续使用洛必达法则, 即 ${\lim }_{x\to a}\frac{f(x)}{F(x)}={\lim }_{x\to a}\frac{f^{\prime }(x)}{F^{\prime }(x)}={\lim }_{x\to a}\frac{f^{\prime \prime }(x)}{F^{\prime \prime }(x)}$.

重要的比大小原材料

(1)当 $x\to +\infty$ 时, 有 ${\ln }^{\alpha }x?x^{\beta }?a^x$, 其中 $\alpha ,\beta >0,a>1$, 符号 “ ?” 叫远远小于;
(2) 当 $n\to \infty$ 时, 有 ${\ln }^{\alpha }n?n^{\beta }?a^n?n!?n^n$, 其中 $\alpha ,\beta >0,a>1$.
题目:
1.13 解 (1) ${\lim }_{x\to +\infty }\frac{\ln x}{x^n}={\lim }_{x\to +\infty }\frac{\frac{1}{x}}{n{x}^{n?1}}={\lim }_{x\to +\infty }\frac{1}{n{x}^n}=0$.
$$\underset{x\to +\infty }{\lim }\frac{x^n}{{\mathrm{e}}^{\lambda x}}=\underset{x\to +\infty }{\lim }\frac{n{x}^{n?1}}{\lambda {\mathrm{e}}^{\lambda x}}=\underset{x\to +\infty }{\lim }\frac{n(n?1)x^{n?2}}{{\lambda }^2{\mathrm{e}}^{\lambda x}}=?=\underset{x\to +\infty }{\lim }\frac{n!}{{\lambda }^n{\mathrm{e}}^{\lambda x}}=0.$$

泰勒公式

$$\begin{array}{ll}\sin x=x?\frac{x^3}{3!}+o\left(x^3\right),& \cos x=1?\frac{x^2}{2!}+\frac{x^4}{4!}+o\left(x^4\right),\\ \arcsin x=x+\frac{x^3}{3!}+o\left(x^3\right),& \tan x=x+\frac{x^3}{3}+o\left(x^3\right),\\ \arctan x=x?\frac{x^3}{3}+o\left(x^3\right),& \ln (1+x)=x?\frac{x^2}{2}+\frac{x^3}{3}+o\left(x^3\right),\\ {\mathrm{e}}^x=1+x+\frac{x^2}{2!}+\frac{x^3}{3!}+o\left(x^3\right),& (1+x{)}^{\alpha }=1+\alpha x+\frac{\alpha (\alpha ?1)}{2!}{x}^2+o\left(x^2\right).\end{array}$$

差函数

$$x?\sin x=\frac{1}{6}{x}^3+o\left(x^3\right),x?\sin x～\frac{1}{6}{x}^3(x\to 0),x?\ln (1+x)～\frac{1}{2}{x}^2(x\to 0)$$
$$\arcsin x?x～\frac{1}{6}{x}^3(x\to 0),\tan x?x～\frac{1}{3}{x}^3(x\to 0),x?\arctan x～\frac{x^3}{3}(x\to 0)$$

无穷小的运算

设 $m,n$ 为正整数, 则

(1) o ( x m ) ± o ( x n ) = o ( x l ) , l = min ? { m , n } o\left(x^{m}\right) \pm o\left(x^{n}\right)=o\left(x^{l}\right), l=\min \{m, n\} o(xm)±o(xn)=o(xl),l=min{m,n} ( 加减法时低阶 “吸收” 高阶)；

(2) $o\left(x^m\right)?o\left(x^n\right)=o\left(x^{m+n}\right),x^m?o\left(x^n\right)=o\left(x^{m+n}\right)$ ( 乘法时阶数 “累加” );

(3) $o\left(x^m\right)=o\left(k{x}^m\right)=k?o\left(x^m\right),k\ne 0$ 且为常数 (非零常数相乘不影响阶数).

泰勒使用两型:

(1) $\frac{A}{B}$ 型，适用 “上下同阶” 原则 .
具体说来, 如果分母 (或分子) 是 $x$ 的 $k$ 次幕, 则应把分子 (或分母) 展开到 $x$ 的 $k$ 次幂, 可称为 “上下同阶”原则 .
例如, 计算 ${\lim }_{x\to 0}\frac{x?\ln (1+x)}{x^2}$.

(2) $A?B$ 型，适用 “幂次最低” 原则 .
具体说来, 即将 $A,B$ 分别展开到它们的系数不相等的 $x$ 的最低次幂为止.
例如, 已知当 $x\to 0$ 时, $\cos x?{\mathrm{e}}^{?\frac{x^2}{2}}$ 与 $a{x}^b$ 为等价无穷小, 求 $a,b$.

两个重要极限

${\lim }_{x\to 0}\frac{\sin x}{x}=1$ 和 ${\lim }_{x\to \infty }{\left(1+\frac{1}{x}\right)}^x=e$

${\lim }_{x\to 0}{\left(1+x\right)}^{\frac{1}{x}}=e$

夹逼准则 适当放缩 $\{\begin{array}{l}\text{?已知不等式?}\\ \text{?题设条件?}\end{array}$

如果函数 $f(x),g(x)$ 及 $h(x)$ 满足下列条件 :

(1) $h(x)?f(x)?g(x)$;

(2) $\lim g(x)=A,\lim h(x)=A$.

则 $\lim f(x)$ 存在, 且 $\lim f(x)=A$.

七种未定式的计算

七种未定式:
$$\frac{0}{0},\frac{\infty }{\infty },0?\infty ,\infty ?\infty ,{\infty }^0,0^0,1^{\infty }$$
(1)化简先行
(2)判断类型 (运算类型)
(3)选择方法（洛必达法则、泰勒公式、夹逼准则等）。

(1) $\frac{0}{0},\frac{\infty }{\infty },0?\infty$.

都是使用无穷小替换,$0?\infty$要转换形式
抓大头:
分母中关于 $x$ 的最高次项, 忽略其他项, 如 ${\lim }_{x\to ?\infty }\frac{\sqrt{4x^2+x?1}+\sqrt{x+1}}{\sqrt{x^2+\sin x}}=1$. 另外特别注意, 若 $x\to 0$, 则应该分别抓分子、分母中关于 $x$ 的最低次项. $|2x|=?2x|x|=?x$
(2) “ $\infty ?\infty ”$.
(1) 如果函数中有分母, 则通分, 将加减法变形为乘除法, 以便于使用其他计算工具 (比如洛必达法则)

(2) 如果函数中没有分母, 则可以通过提取公因式或者作倒代换，出现分母后，再利用通分等恒等变形的方法, 将加减法变形为乘除法, 见例

(3)$1^{\infty }$
1.3 设函数 $f(x)=\frac{1}{{\mathrm{e}}^{\frac{x}{x?1}}?1}$, 则 $()$.

(A) $x=0,x=1$ 都是 $f(x)$ 的第一类间断点

(B) $x=0,x=1$ 都是 $f(x)$ 的第二类间断点

(C) $x=0$ 是 $f(x)$ 的第一类间断点, $x=1$ 是 $f(x)$ 的第二类间断点

(D) $x=0$ 是 $f(x)$ 的第二类间断点, $x=1$ 是 $f(x)$ 的第一类间断点

函数的连续与间断 讨论间断点 $\{\begin{array}{l}\text{?无定义点?(必间断)?}\\ \text{?分段点?(未必间断)?}\end{array}$

连续点的定义

$$\underset{x\to x_0^{+}}{\lim }f(x)=\underset{x\to x_0^{?}}{\lim }f(x)=f\left(x_0\right)?f(x)\text{?在点?}{x}_0\text{?处连续.?}$$

连续性运算法则

(1) (连续性的四则运算法则) 设 $f(x)$ 与 $g(x)$ 都在点 $x=x_0$ 处连续,
则 $f(x)\pm g(x)$ 与 $f(x)g(x)$ 在点 $x=x_0$ 处连续,
当 $g\left(x_0\right)\ne 0$ 时, $f(x)/g(x)$ 在点 $x=x_0$ 处也连续.

(2) (复合函数的连续性) 设 $u=\phi (x)$ 在点 $x=x_0$ 处连续
$y=f(u)$ 在点 $u=u_0$ 处连续, 且 $u_0=\phi \left(x_0\right)$,
则 $f[\phi (x)]$ 在点 $x=x_0$ 处连续.

(3) (反函数的连续性) 设 $y=f(x)$ 在区间 $I_x$ 上单调且连续,
则反函数 $x=\phi (y)$ 在对应的区间 I y = { y ∣ y = f ( x ) , x ∈ I x } I_{y}=\left\{y \mid y=f(x), x \in I_{x}\right\} Iy?={y∣y=f(x),x∈Ix?} 上连续且有相同的单调性.

间断点的定义与分类

(1) 可去间断点 .
若 ${\lim }_{x\to x_0}f(x)=A\ne f\left(x_0\right)(f\left(x_0\right)$ 甚至可以无定义 ), 则 $x=x_0$ 称为可去间断点.
(2) 跳跃间断点 .
若 ${\lim }_{x\to x_0^{?}}f(x)$ 与 ${\lim }_{x\to x_0^{+}}f(x)$ 都存在, 但 ${\lim }_{x\to x_0^{?}}f(x)\ne {\lim }_{x\to x_0^{+}}f(x)$, 则 $x=x_0$ 称为跳跃间断点.

可去间断点和跳跃间断点统称为第一类间断点．
(3) 无穷间断点 .
若 ${\lim }_{x\to x_0}f(x)=\infty$ 或 ${\lim }_{x\to x_0^{+}}f(x)=\infty$ 或 ${\lim }_{x\to x_0^{?}}f(x)=\infty$, 则 $x=x_0$ 称为无穷间断点, 如点 $x=0$ 为函数 $y=\frac{1}{x}$的无穷间断点 .
(4) 振荡间断点 .
若 ${\lim }_{x\to x_0}f(x)$ 振荡不存在,则 $x=x_0$ 称为振荡间断点,
如函数 $y=\sin \frac{1}{x}$ 在点 $x=0$ 处没有定义, 且当 $x\to 0$ 时, 函数值在 -1 与 1 这两个数之间交替振荡取值, 极限不存在, 故点 $x=0$ 为函数 $y=\sin \frac{1}{x}$ 的振荡间断点 .

无穷间断点和振荡间断点都属于第二类间断点.
题目:
1.2 设 $f(x)$ 在 $(?\infty ,+\infty )$ 内有定义, 且 ${\lim }_{x\to \infty }f(x)=a,g(x)=\{\begin{array}{ll}f\left(\frac{1}{x}\right),& x\ne 0,\\ 0,& x=0,\end{array}$ 则 $()$.

(A) $x=0$ 必是 $g(x)$ 的第一类间断点

(B) $x=0$ 必是 $g(x)$ 的第二类间断点

(C) $x=0$ 必是 $g(x)$ 的连续点

(D) $g(x)$ 在点 $x=0$ 处的连续性与 $a$ 的取值有关

1.3 设函数 $f(x)=\frac{1}{{\mathrm{e}}^{\frac{x}{x?1}}?1}$, 则 $()$.

(A) $x=0,x=1$ 都是 $f(x)$ 的第一类间断点

(B) $x=0,x=1$ 都是 $f(x)$ 的第二类间断点

(C) $x=0$ 是 $f(x)$ 的第一类间断点, $x=1$ 是 $f(x)$ 的第二类间断点

(D) $x=0$ 是 $f(x)$ 的第二类间断点, $x=1$ 是 $f(x)$ 的第一类间断点

1.4 函数 $f(x)={\lim }_{t\to 0}{\left(1+\frac{\sin t}{x}\right)}^{\frac{x^2}{t}}$ 在 $(?\infty ,+\infty )$ 内 $()$.
(A) 连续
(B) 有可去间断点
(C) 有跳跃间断点
(D) 有无穷间断点

1.5 设函数 $f(x)={\lim }_{n\to \infty }\frac{1+x}{1+x^{2n}}$, 关于该函数的间断点, 下列结论正确的是（）.
(A) 不存在间断点
(B) 存在间断点 $x=1$
(C) 存在间断点 $x=0$
(D) 存在间断点 $x=?1$