leetcode刷题记录07(2023-04-30)【二叉树展开为链表 | 买卖股票的最佳时机 | 二叉树中的最大路径和(递归) | 最长连续序列(并查集)】
114. 二叉树展开为链表
给你二叉树的根结点 root ,请你将它展开为一个单链表:
展开后的单链表应该同样使用 TreeNode ,其中 right 子指针指向链表中下一个结点,而左子指针始终为 null 。
展开后的单链表应该与二叉树 先序遍历 顺序相同。
示例 1:

输入:root = [1,2,5,3,4,null,6]
输出:[1,null,2,null,3,null,4,null,5,null,6]
示例 2:
输入:root = []
输出:[]
示例 3:
输入:root = [0]
输出:[0]
提示:
树中结点数在范围
[
0
,
2000
]
内
树中结点数在范围 [0, 2000] 内
树中结点数在范围[0,2000]内
?
100
<
=
N
o
d
e
.
v
a
l
<
=
100
-100 <= Node.val <= 100
?100<=Node.val<=100
进阶:你可以使用原地算法(O(1) 额外空间)展开这棵树吗?
现在刷leetcode经常一遍就可以通过,代码不需要调试,感觉还是很有成就感的,刷题确实有效果。
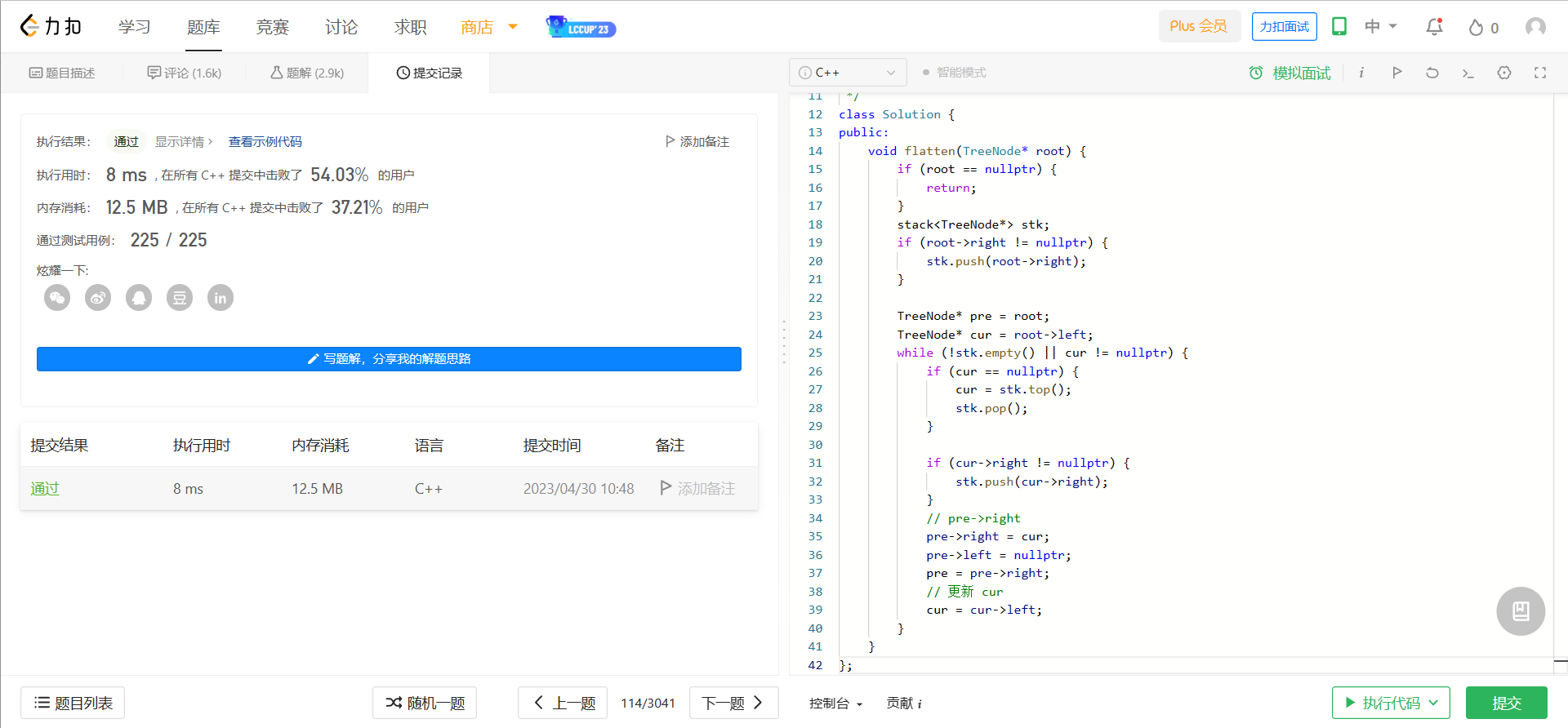
这道题目如果不要求使用原地算法(O(1) 额外空间),很简单,进行前序遍历,保存数组的指针到数组中,最后再进行一次树的重建,但是复杂度是O(n)。
或者采用一个栈的数据结构,保存一下右子树,不断地进行前序遍历,将当前节点添加到 pre->right 中,pre->left 中填写 nullptr。
值得注意的是,需要对空子树进行处理,如果 root 节点为空,直接返回。
代码如下:
#include<stack>
using namespace std;
// Definition for a binary tree node.
struct TreeNode {
int val;
TreeNode* left;
TreeNode* right;
TreeNode() : val(0), left(nullptr), right(nullptr) {}
TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
TreeNode(int x, TreeNode* left, TreeNode* right) : val(x), left(left), right(right) {}
};
class Solution {
public:
void flatten(TreeNode* root) {
if (root == nullptr) {
return;
}
stack<TreeNode*> stk;
if (root->right != nullptr) {
stk.push(root->right);
}
// 前驱节点
TreeNode* pre = root;
// 当前节点
TreeNode* cur = root->left;
while (!stk.empty() || cur != nullptr) {
if (cur == nullptr) {
cur = stk.top();
stk.pop();
}
// 将右节点入栈
if (cur->right != nullptr) {
stk.push(cur->right);
}
// pre->right
pre->right = cur;
pre->left = nullptr;
pre = pre->right;
// 更新 cur
cur = cur->left;
}
}
};
虽然采用栈降低了空间复杂度,但是也并不能说是O(1)级别的,看了下题解,思路是:对于每个节点,每次将它的左节点变为空,然后就移动到它的右节点上去。主要是找到左子树中最后一个访问的节点,将右子树移到它上面,最后再将左子树整体移动到 root->right 上。
代码如下:
class Solution {
public:
void flatten(TreeNode* root) {
TreeNode *curr = root;
while (curr != nullptr) {
if (curr->left != nullptr) {
auto next = curr->left;
auto predecessor = next;
while (predecessor->right != nullptr) {
predecessor = predecessor->right;
}
predecessor->right = curr->right;
curr->left = nullptr;
curr->right = next;
}
curr = curr->right;
}
}
};
121. 买卖股票的最佳时机
给定一个数组 prices ,它的第 i 个元素 prices[i] 表示一支给定股票第 i 天的价格。
你只能选择 某一天 买入这只股票,并选择在 未来的某一个不同的日子 卖出该股票。设计一个算法来计算你所能获取的最大利润。
返回你可以从这笔交易中获取的最大利润。如果你不能获取任何利润,返回 0 。
示例 1:
输入:[7,1,5,3,6,4]
输出:5
解释:在第 2 天(股票价格 = 1)的时候买入,在第 5 天(股票价格 = 6)的时候卖出,最大利润 = 6-1 = 5 。
注意利润不能是 7-1 = 6, 因为卖出价格需要大于买入价格;同时,你不能在买入前卖出股票。
示例 2:
输入:prices = [7,6,4,3,1]
输出:0
解释:在这种情况下, 没有交易完成, 所以最大利润为 0。
提示:
1
<
=
p
r
i
c
e
s
.
l
e
n
g
t
h
<
=
1
0
5
1 <= prices.length <= 10^5
1<=prices.length<=105
0
<
=
p
r
i
c
e
s
[
i
]
<
=
1
0
4
0 <= prices[i] <= 10^4
0<=prices[i]<=104
还是一遍过!

主要思路就是从前向后进行遍历,保存最小值,然后用当前值-最小值,如果大于当前的最大利润,就更新最大利润。最后返回最大利润。
代码如下:
#include<vector>
using namespace std;
class Solution {
public:
int maxProfit(vector<int>& prices) {
int minVal = 0x3f3f3f3f;
int profit = 0;
for (int i = 0; i < prices.size(); i++) {
if (prices[i] < minVal)minVal = prices[i];
profit = profit > (prices[i] - minVal) ? profit : (prices[i] - minVal);
}
return profit;
}
};
124. 二叉树中的最大路径和
二叉树中的 路径 被定义为一条节点序列,序列中每对相邻节点之间都存在一条边。同一个节点在一条路径序列中 至多出现一次 。该路径 至少包含一个 节点,且不一定经过根节点。
路径和 是路径中各节点值的总和。
给你一个二叉树的根节点 root ,返回其 最大路径和 。
示例 1:
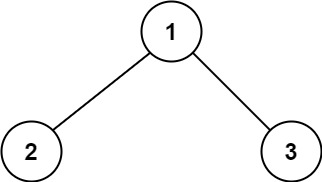
输入:root = [1,2,3]
输出:6
解释:最优路径是 2 -> 1 -> 3 ,路径和为 2 + 1 + 3 = 6
示例 2:
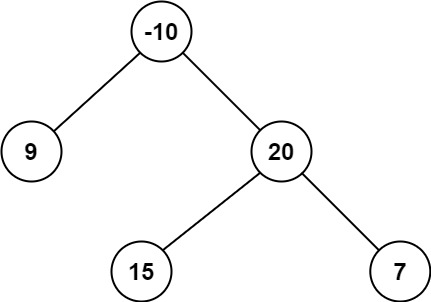
输入:root = [-10,9,20,null,null,15,7]
输出:42
解释:最优路径是 15 -> 20 -> 7 ,路径和为 15 + 20 + 7 = 42
提示:
树中节点数目范围是
[
1
,
3
?
1
0
4
]
树中节点数目范围是 [1, 3 * 10^4]
树中节点数目范围是[1,3?104]
?
1000
<
=
N
o
d
e
.
v
a
l
<
=
1000
-1000 <= Node.val <= 1000
?1000<=Node.val<=1000
很优美的代码,主要通过一个共享成员变量记录全局最优解,再 maxGain 内部有进行局部最优解的计算,并不断更新全局最优解。
同时,maxGain 的返回值,返回的是当前节点作为路径的一部分,要么要左子树,要么要右子树,不能两个都要(这样才能保证 同一个节点在一条路径序列中至多出现一次 )。
#include<algorithm>
using namespace std;
// Definition for a binary tree node.
struct TreeNode {
int val;
TreeNode *left;
TreeNode *right;
TreeNode() : val(0), left(nullptr), right(nullptr) {}
TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
};
class Solution {
int maxSum = -0x3f3f3f3f - 1;
int maxGain(TreeNode* root) {
if (root == nullptr) {
return 0;
}
int leftGain = max(maxGain(root->left), 0);
int rightGain = max(maxGain(root->right), 0);
int curMax = root->val + leftGain + rightGain;
maxSum = max(maxSum, curMax);
return root->val + max(leftGain, rightGain);
}
public:
int maxPathSum(TreeNode* root) {
maxGain(root);
return maxSum;
}
};
128. 最长连续序列
给定一个未排序的整数数组 nums ,找出数字连续的最长序列(不要求序列元素在原数组中连续)的长度。
请你设计并实现时间复杂度为 O(n) 的算法解决此问题。
示例 1:
输入:nums = [100,4,200,1,3,2]
输出:4
解释:最长数字连续序列是 [1, 2, 3, 4]。它的长度为 4。
示例 2:
输入:nums = [0,3,7,2,5,8,4,6,0,1]
输出:9
提示:
0
<
=
n
u
m
s
.
l
e
n
g
t
h
<
=
1
0
5
0 <= nums.length <= 10^5
0<=nums.length<=105
?
1
0
9
<
=
n
u
m
s
[
i
]
<
=
1
0
9
-10^9 <= nums[i] <= 10^9
?109<=nums[i]<=109
主要思路就是采用并查集来实现,首先要去重,得到一个哈希表,然后遍历这个哈希,如果存在连续的数,那么就需要进行合并,合并的时候要将大的数作为根,这样我们就将所有连续的数划分在一个集合里面了。
最后遍历一遍哈希表,判断当前元素是否为序列的第一个数。
- 如果是第一个数,则找到它的祖先,根据祖先的值,就可以确定序列的长度。
- 如果不是第一个数,不考虑。
#include<vector>
#include<unordered_map>
using namespace std;
class Solution {
vector<int> mergeAndFindSet;
int find(int val) {
while (mergeAndFindSet[val] != val)
{
val = mergeAndFindSet[val];
}
return val;
}
void merge(int val1, int val2) {
int i1 = find(val1);
int i2 = find(val2);
// 找到 i1 和 i2 后进行合并
if (i1 == i2) return;
mergeAndFindSet[i1] = i2;
}
public:
int longestConsecutive(vector<int>& nums) {
unordered_map<int, int> mp;
int n = nums.size();
mergeAndFindSet = vector<int>(n, 0);
for (int i = 0; i < n; i++) {
mergeAndFindSet[i] = i;
}
for (int i = 0; i < n; i++) {
mp[nums[i]] = i;
}
for (auto it = mp.begin(); it != mp.end(); it++) {
int num = it->first;
int i = it->second;
if (mp.count(num + 1)) { // 如果有连续的数就进行合并
merge(i, mp[num + 1]); // 合并需要将小的数合并到最大的根上
}
}
int res = 0;
for (auto it = mp.begin(); it != mp.end(); it++) {
int num = it->first;
int i = it->second;
if (!mp.count(num - 1)) { // 如果当前这个数是最小的,寻找它的根
int root = find(i);
res = max(res, nums[root] - num + 1);
}
}
return res;
}
};
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:chenni525@qq.com进行投诉反馈,一经查实,立即删除!