优先级队列(堆) PriorityQueue

• 🎥?个人主页：Dikz12
• 📕格言：那些在暗处执拗生长的花，终有一日会馥郁传香
• 欢迎大家👍点赞?评论?收藏

目录

?1.优先级队列?

2.优先级队列的模拟实现

2.1 堆的概念

2.2 堆的创建?

2.3 堆的插入和删除?

2.4 建堆的时间复杂度

?3.PriorityQueue接口介绍

---

?1.优先级队列?

? ?有些情况下，操作的数据可能带有优先级，一般出队列时，可能需要优先级高的元素先出队列，该中场景下，使用队列显然不合适，比如：初中那会班主任排座位时可能会让成绩好的同学先挑座位。

2.优先级队列的模拟实现

优先级队列 （?Priority Queue）底层使用了堆这种数据结构，而堆实际上就是对完全二叉树进行了一些调整。

2.1 堆的概念

?堆的性质：

• 堆中某个节点的值总是不大于或不小于其父(根)节点的值；
• ?堆是一颗完全二叉树

?所以，堆可以分为大根堆和小根堆，也都是完全二叉树。

• ?小根堆：根节点 比 左右孩子都小；左右孩子谁最小没有关系，只考虑根和左右孩子的关系

• 大根堆：?根节点 比 左右孩子都大

2.2 堆的创建?

?对于集合{ 27,15,19,18,28,34,65,49,25,37 }中的数据，如果将其创建成堆呢？

?可以采用向下调整方式，创建大/小根堆：

创建大根堆 ：

?整个调整过程：

1.从最后一颗子树开始调整

2.找到左右孩子的最大值 和 根节点进行比较，如果比根节点大，那么就交换

3.如果能够知道子树的根节点下标，那么下一颗子树的位置就是当前根节点下标 -1

4.一直调整到下标为0结束

关键问题：

1.每棵子树调整的时候，什么时候结束?

? 就是孩子的下标要小于length

2.最后一颗子树的根节点下标如何确定？

(9-1) / 2 => (length -1) / 2

    public void createBigHeap() {
        for (int parent = (usedSize - 1 - 1) / 2; parent >= 0; parent--) {
            siftDown(parent,usedSize);
        }
    }
    public void siftDown(int parent, int end) {

        int child = parent * 2 + 1;
        while (child < end) {
            if (child + 1 < usedSize && elem[child] < elem[child + 1]) {
                child++;
            }
            //开始调整 此时child下标，一定是最大值
            if (elem[child] > elem[parent]) {
                //交换
                swap(child, parent);
                //交换之后，验证下面是否还满足大根堆
                parent = child;
                child = parent * 2 + 1;
            } else {
                //都满足
                break;
            }
        }
    }
    public void swap(int i,int j) {
        int tmp = elem[i];
        elem[i] = elem[j];
        elem[j] = tmp;
    }

2.3 堆的插入和删除?

?插入:

?

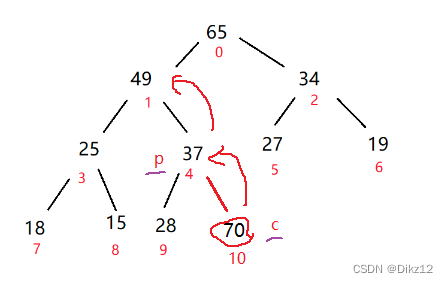
1.判断是否需要扩容

2.把插入的值放到最后

3.开始向上调整

关键问题：

?什么时候调整结束？

4? 到---> 1? : （p?- 1）/ 2 ,在 c = p，?由此推-> 当 c == 0 或者 p < 0 结束?。

插入后：

    //插入
    public void offer(int val) {
        //1.是否需要扩容
        if (isFull()) {
            this.elem = Arrays.copyOf(elem,2*elem.length);
        }
        //2.向上调整
        elem[usedSize] = val;
        siftUp(usedSize);
        usedSize++;
    }
    public void siftUp(int child) {
        int parent = (child - 1) / 2;
        while (child > 0) {
            if (elem[child] > elem[parent]) {
                swap(child,parent);
                child = parent;
                parent = (child - 1) / 2;
            } else {
                break;
            }
        }
    }
    public boolean isFull() {
        return usedSize == elem.length;
    }

删除：

1.交换0 和 最后一个下标的值，uesdSize--

2.在向下调整就行

    //删除
    public int poll() {
        if (empty()) {
            return -1;
        }
        int delVal = elem[0];
        swap(0,usedSize-1);
        usedSize--;
        siftDown(0,usedSize);
        return delVal;
    }
    public boolean empty() {
        return usedSize == 0;
    }

2.4 建堆的时间复杂度

因此，堆向下调整建堆的时间复杂度为O(n)。

?3.PriorityQueue接口介绍

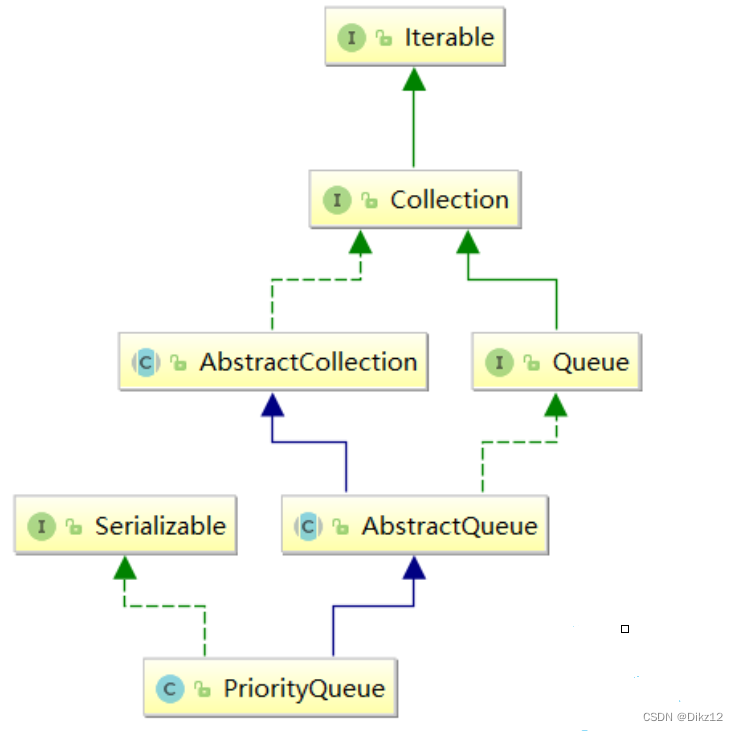
?注意：

1. 使用时必须导入PriorityQueue所在的包，即：

import java.util.PriorityQueue;

2. PriorityQueue中放置的元素必须要能够比较大小，不能插入无法比较大小的对象，否则会抛出
ClassCastException异常。
3. 不能插入null对象，否则会抛出NullPointerException。
4. 没有容量限制，可以插入任意多个元素，其内部可以自动扩容。
5. 插入和删除元素的时间复杂度为O(log2N)。
6. PriorityQueue底层使用了堆数据结构。
7. PriorityQueue默认情况下是小堆---即每次获取到的元素都是最小的元素。
?