[足式机器人]Part4 南科大高等机器人控制课 Ch08 Rigid Body Dynamics

本文仅供学习使用
本文参考：
B站：CLEAR_LAB
笔者带更新-运动学
课程主讲教师：
Prof. Wei Zhang

---

1. Spatial Vecocity

Given a rigid body with spatial velocity $\mathcal{V}=\left(\vec{\omega },\vec{v}\right)$ , its spatial acceleration (coordinate-free)
$$\mathcal{A}=\dot{\mathcal{V}}=\left[\begin{array}{c}\dot{\vec{\omega }}\\ {\dot{\vec{v}}}_{\mathrm{O}}\end{array}\right],\mathcal{A}=\underset{\delta \to 0}{\lim }\frac{\mathcal{V}\left(t+\delta \right)?\mathcal{V}\left(t\right)}{\delta }$$
Recall that: ${\vec{v}}_{\mathrm{O}}$ i sthe velocity of the body-fixed particle coincident with frame origin $o$ at the current time $t$

Note : $\dot{\vec{\omega }}$ is the angular acceleration of the body

${\dot{\vec{v}}}_{\mathrm{O}}$ is not the acceleration of any body-fixed point ! ${\vec{v}}_{\mathrm{O}}={\dot{\vec{R}}}_q\left(t\right),{\dot{\vec{v}}}_{\mathrm{O}}\ne {\ddot{\vec{R}}}_q\left(t\right)$
In face, ${\dot{\vec{v}}}_{\mathrm{O}}$ gives the rate of change in stream velocity of body-fixed particles passing through $o$

1.1 Spatial vs. Conventional Accel

Suppose ${\vec{R}}_q\left(t\right)$ is the body fixed particle coincides with $o$ at time $t$
So by definition , we have ${\vec{v}}_{\mathrm{O}}\left(t\right)={\dot{\vec{R}}}_q\left(t\right)$ , however ${\dot{\vec{v}}}_{\mathrm{O}}\ne {\ddot{\vec{R}}}_q\left(t\right)$ , where ${\ddot{\vec{R}}}_q\left(t\right)$ is the conventional acceleration of the body-fixed point $q$

At time $t$ : ${\vec{R}}_q\left(t\right)=0$ , ${\vec{v}}_{\mathrm{O}}\left(t\right)={\dot{\vec{R}}}_q\left(t\right)$
At time $t+\delta$ : ${\vec{R}}_{q^{\prime }}\left(t\right)=0$ , ${\vec{v}}_{\mathrm{O}}\left(t+\delta \right)={\dot{\vec{R}}}_{q^{\prime }}\left(t+\delta \right)\ne {\dot{\vec{R}}}_q\left(t+\delta \right)$ —— $q^{\prime }$ another body-fixed particle

• Note : $q$ and $q^{\prime }$ are different points, $\underset{\delta \to 0}{\lim }{\vec{v}}_{\mathrm{O}}\left(t\right)=\frac{{\vec{v}}_{\mathrm{O}}\left(t+\delta \right)?{\vec{v}}_{\mathrm{O}}\left(t\right)}{\delta }=\frac{{\dot{\vec{R}}}_{q^{\prime }}\left(t+\delta \right)?{\vec{R}}_q\left(t\right)}{\delta }$

实际上只需考虑Twist最开始的定义，即速度 ${\vec{v}}_{\mathrm{O}}$ 并不是某一点的速度，而是考虑相对坐标系原点而言的虚拟点在该角速度下的瞬时速度（${\dot{\vec{R}}}_q\left(t\right)={\vec{v}}_{\mathrm{O}}\left(t\right)+\vec{\omega }\left(t\right)\times {\vec{R}}_q\left(t\right)$），而与该坐标系所代表的真实点的运动无关(${\vec{R}}_q\left(t\right)$ is the body fixed particle coincides with $o$ at time $t$)，即为：
$${\ddot{\vec{R}}}_q\left(t\right)={\dot{\vec{v}}}_{\mathrm{O}}\left(t\right)+\dot{\vec{\omega }}\left(t\right)\times {\vec{R}}_q{\left(t\right)}_{\nearrow 0}+\vec{\omega }\left(t\right)\times {\dot{\vec{R}}}_q\left(t\right)={\dot{\vec{v}}}_{\mathrm{O}}\left(t\right)+\vec{\omega }\left(t\right)\times {\dot{\vec{R}}}_q\left(t\right)$$

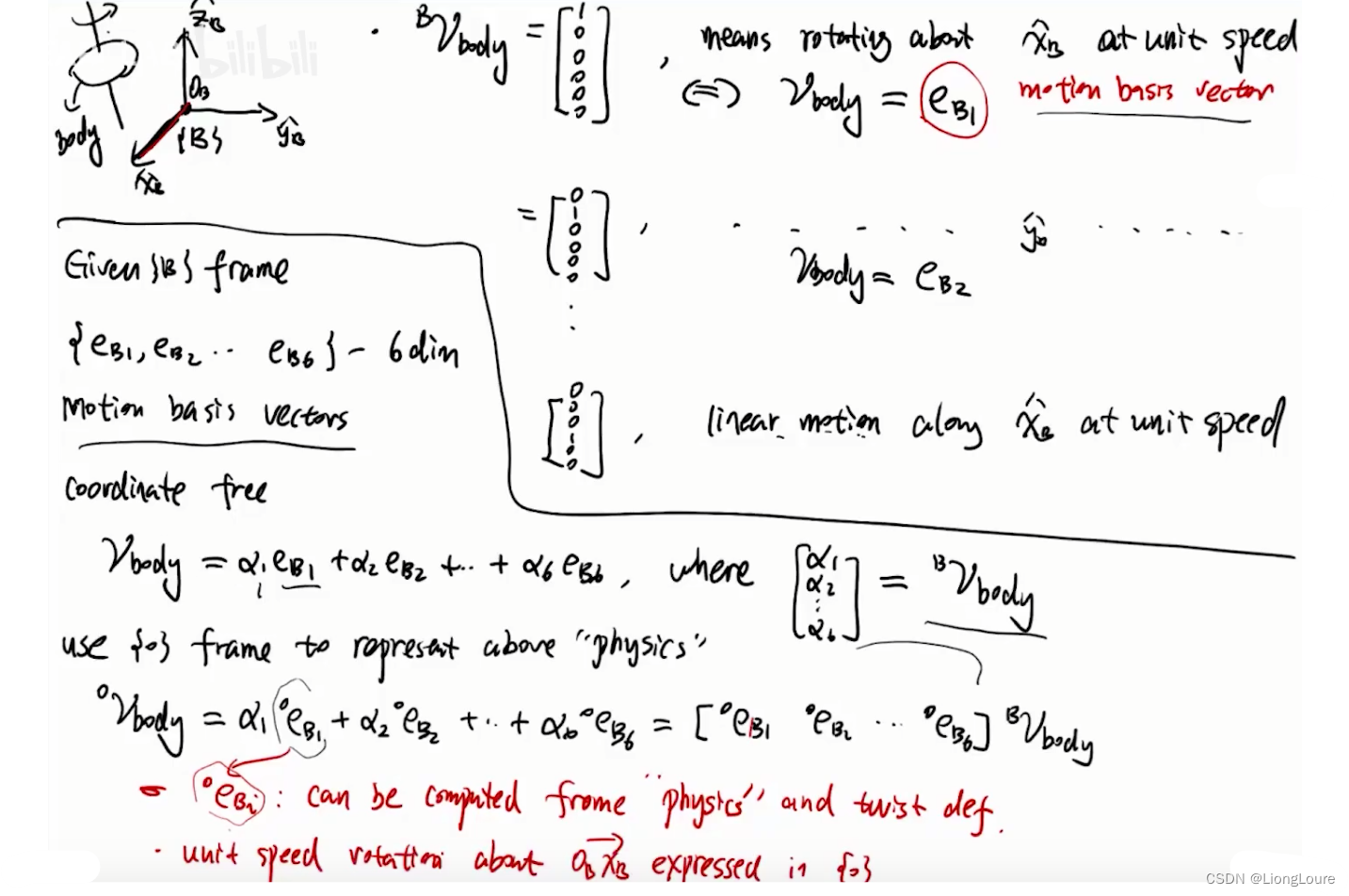
1.2 Plueker Coordinate System and Basis Vectors

按照向量的本质理解即可，这也是笔者为啥不是很喜欢旋量的原因。

Recall coordinate-free concept: let $\vec{R}\in {\mathbb{R}}^3$ be a free vector with { O } \left\{ O \right\} {O} and { B } \left\{ B \right\} {B} frame coordinate ${\vec{R}}^O$ and ${\vec{R}}^B$

矢量的变换：

旋量的变换：

$${\left[\begin{array}{lccccc}{e}_{\mathrm{B}1}^O& e_{\mathrm{B}2}^O& e_{\mathrm{B}3}^O& e_{\mathrm{B}4}^O& e_{\mathrm{B}4}^O& e_{\mathrm{B}5}^O\end{array}\right]}_{6\times 6}=\left[X_{\mathrm{B}}^O\right]=\left[A{d}_{\left[T_{\mathrm{B}}^O\right]}\right]$$

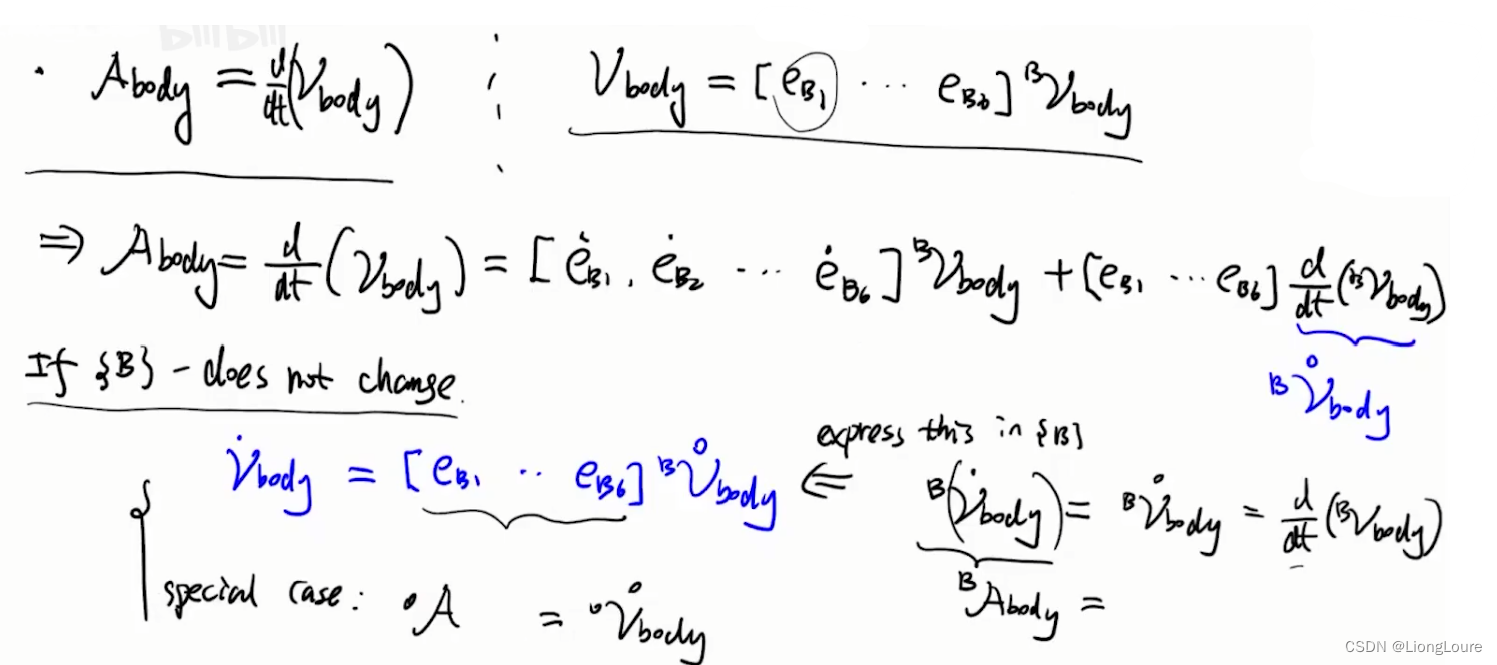
1.3 Work with Moving Reference Frame

Now let’s work with { O } \left\{ O \right\} {O} frame to find the derivative —— we need to compute :${\left[\begin{array}{lccccc}{\dot{e}}_{\mathrm{B}1}^O& {\dot{e}}_{\mathrm{B}2}^O& {\dot{e}}_{\mathrm{B}3}^O& {\dot{e}}_{\mathrm{B}4}^O& {\dot{e}}_{\mathrm{B}4}^O& {\dot{e}}_{\mathrm{B}5}^O\end{array}\right]}_{6\times 6}=\left[{\dot{X}}_{\mathrm{B}}^O\right]=\frac{\mathrm{d}}{\mathrm{d}t}\left[A{d}_{\left[T_{\mathrm{B}}^O\right]}\right]$

Let’s denote : $\left[T_{\mathrm{B}}^O\right]=\left(\left[Q\right],\vec{R}\right)?\frac{\mathrm{d}}{\mathrm{d}t}\left(\left[\begin{array}{cc}\left[Q\right]& 0\\ \widetilde{\stackrel{⃗}{R}}\left[Q\right]& \left[Q\right]\end{array}\right]\right)=\left[\begin{array}{cc}\left[\dot{Q}\right]& 0\\ {\left(\widetilde{\stackrel{⃗}{R}}\left[Q\right]\right)}^{\prime }& \left[\dot{Q}\right]\end{array}\right]$

{ B } \left\{ B \right\} {B} frame has instantaneous velocity ${\mathcal{V}}_B=\left[\begin{array}{c}\vec{\omega }\\ {\vec{v}}_{\mathrm{O}}\end{array}\right]$

1.4 Derivative of Adjoint

Note : $\left[\dot{Q}\right]=\vec{\omega }\times \left[Q\right],\dot{\vec{R}}={\vec{v}}_{\mathrm{O}}+\vec{\omega }\times \vec{R},\widetilde{\left[Q\right]\vec{\omega }}=\left[Q\right]\widetilde{\stackrel{⃗}{\omega }}{\left[Q\right]}^{\mathrm{T}},\widetilde{{\vec{\omega }}_1\times {\vec{\omega }}_2}={\widetilde{\stackrel{⃗}{\omega }}}_1{\widetilde{\stackrel{⃗}{\omega }}}_2?{\widetilde{\stackrel{⃗}{\omega }}}_2{\widetilde{\stackrel{⃗}{\omega }}}_1$(Jacobi’s Identity)

After some computation :
$$\frac{\mathrm{d}}{\mathrm{d}t}\left[A{d}_{\left[T_{\mathrm{B}}^O\right]}\right]=\left[\begin{array}{cc}\widetilde{\stackrel{⃗}{\omega }}& 0\\ {\widetilde{\stackrel{⃗}{v}}}_{\mathrm{O}}& \widetilde{\stackrel{⃗}{\omega }}\end{array}\right]\left[A{d}_{\left[T_{\mathrm{B}}^O\right]}\right]=\left[{\dot{X}}_{\mathrm{B}}^O\right]$$

Define : $\left[\begin{array}{cc}\widetilde{\stackrel{⃗}{\omega }}& 0\\ {\widetilde{\stackrel{⃗}{v}}}_{\mathrm{O}}& \widetilde{\stackrel{⃗}{\omega }}\end{array}\right]={\tilde{\mathcal{V}}}_B$
$$?\begin{array}{l}\left[{\dot{Q}}_{\mathrm{B}}^O\right]={\widetilde{\stackrel{⃗}{\omega }}}_B\left[Q_{\mathrm{B}}^O\right]\\ \left[{\dot{X}}_{\mathrm{B}}^O\right]={\tilde{\mathcal{V}}}_B\left[{\dot{X}}_{\mathrm{B}}^O\right]\end{array}$$
In coordinate free: ${\dot{e}}_{\mathrm{B}1}^O={\tilde{\mathcal{V}}}_B{e}_{\mathrm{B}1}^O$

1.4.1 Spatial Cross Product

Given two spatial velocities(twists) ${\mathcal{V}}_1$ and ${\mathcal{V}}_2$ , their spatial product is
$${\mathcal{V}}_1\times {\mathcal{V}}_2=\left[\begin{array}{c}{\vec{\omega }}_1\\ {{\vec{v}}_1}_{\mathrm{O}}\end{array}\right]\times \left[\begin{array}{c}{\vec{\omega }}_2\\ {{\vec{v}}_2}_{\mathrm{O}}\end{array}\right]=\left[\begin{array}{c}{\vec{\omega }}_1\times {\vec{\omega }}_2\\ {\vec{\omega }}_1\times {{\vec{v}}_2}_{\mathrm{O}}+{{\vec{v}}_1}_{\mathrm{O}}\times {\vec{\omega }}_2\end{array}\right]$$

Matrix representation : ${\mathcal{V}}_1\times {\mathcal{V}}_2={\tilde{\mathcal{V}}}_1{\mathcal{V}}_2,{\tilde{\mathcal{V}}}_1=\left[\begin{array}{cc}{\widetilde{\stackrel{⃗}{\omega }}}_1& 0\\ {{\widetilde{\stackrel{⃗}{v}}}_1}_{\mathrm{O}}& {\widetilde{\stackrel{⃗}{\omega }}}_1\end{array}\right]$

Roughly speaking, when a motion $\mathcal{V}$ is moving with a spatial velocity $\mathcal{Z}$ (e.g. it is attached to a moving frame) but is otherwise not changing , then
$$\dot{\mathcal{V}}=\mathcal{Z}\times \mathcal{V}$$

• Propertries

Assume A is moving wrt $O$ with velocity ${\mathcal{V}}_{\mathrm{A}}$ : $\left[{\dot{X}}_{\mathrm{A}}^O\right]={\tilde{\mathcal{V}}}_{\mathrm{A}}^O\left[X_{\mathrm{A}}^O\right]$
$\widetilde{\left[X\right]\mathcal{V}}=\left[X\right]\tilde{\mathcal{V}}{\left[X\right]}^{\mathrm{T}}$ for any transformation $\left[X\right]$ and twist $\mathcal{V}$

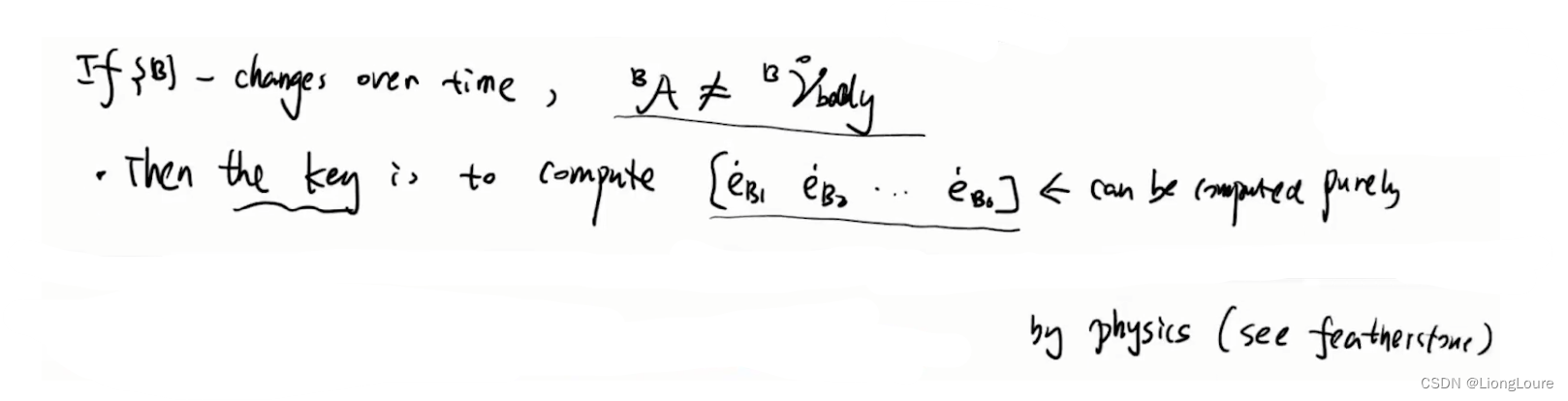
1.4.2 Spatial Acceleration with Moving Reference Frame

Consider a body with velocity ${\mathcal{V}}_{\mathrm{Body}}$ (wrt inertia frame), and ${\mathcal{V}}_{\mathrm{Body}}^O$ and ${\mathcal{V}}_{\mathrm{Body}}^B$ be its Plueker coordinates wrt { O } \left\{ O \right\} {O} and { B } \left\{ B \right\} {B} :
$${\mathcal{A}}_{\mathrm{Body}}^B=\frac{\mathrm{d}}{\mathrm{d}t}\left({\mathcal{V}}_{\mathrm{Body}}^B\right)+{\tilde{\mathcal{V}}}_{\mathrm{BO}}^B{\mathcal{V}}_{\mathrm{Body}}^B$$
$${\mathcal{A}}_{\mathrm{Body}}^O=\left[X_{\mathrm{B}}^O\right]{\mathcal{A}}_{\mathrm{Body}}^B$$

${\mathcal{A}}_{\mathrm{Body}}^O=\frac{\mathrm{d}}{\mathrm{d}t}\left({\mathcal{V}}_{\mathrm{Body}}^O\right)=\frac{\mathrm{d}}{\mathrm{d}t}\left(\left[X_{\mathrm{B}}^O\right]{\mathcal{V}}_{\mathrm{Body}}^B\right)=\left[{\dot{X}}_{\mathrm{B}}^O\right]{\mathcal{V}}_{\mathrm{Body}}^B+\left[X_{\mathrm{B}}^O\right]{\dot{\mathcal{V}}}_{\mathrm{Body}}^B={\tilde{\mathcal{V}}}_{\mathrm{B}}^O\left[X_{\mathrm{B}}^O\right]{\mathcal{V}}_{\mathrm{Body}}^B+\left[X_{\mathrm{B}}^O\right]{\dot{\mathcal{V}}}_{\mathrm{Body}}^B=\left[X_{\mathrm{B}}^O\right]\left(\left[X_{\mathrm{O}}^B\right]{\tilde{\mathcal{V}}}_{\mathrm{B}}^O\left[X_{\mathrm{B}}^O\right]{\mathcal{V}}_{\mathrm{Body}}^B+{\dot{\mathcal{V}}}_{\mathrm{Body}}^B\right)=\left[X_{\mathrm{B}}^O\right]\left(\widetilde{\left[X_{\mathrm{O}}^B\right]{\mathcal{V}}_{\mathrm{B}}^O}{\mathcal{V}}_{\mathrm{Body}}^B+{\dot{\mathcal{V}}}_{\mathrm{Body}}^B\right)=\left[X_{\mathrm{B}}^O\right]\left({\tilde{\mathcal{V}}}_{\mathrm{BO}}^B{\mathcal{V}}_{\mathrm{Body}}^B+{\dot{\mathcal{V}}}_{\mathrm{Body}}^B\right)=\left[X_{\mathrm{B}}^O\right]{\mathcal{A}}_{\mathrm{Body}}^B$

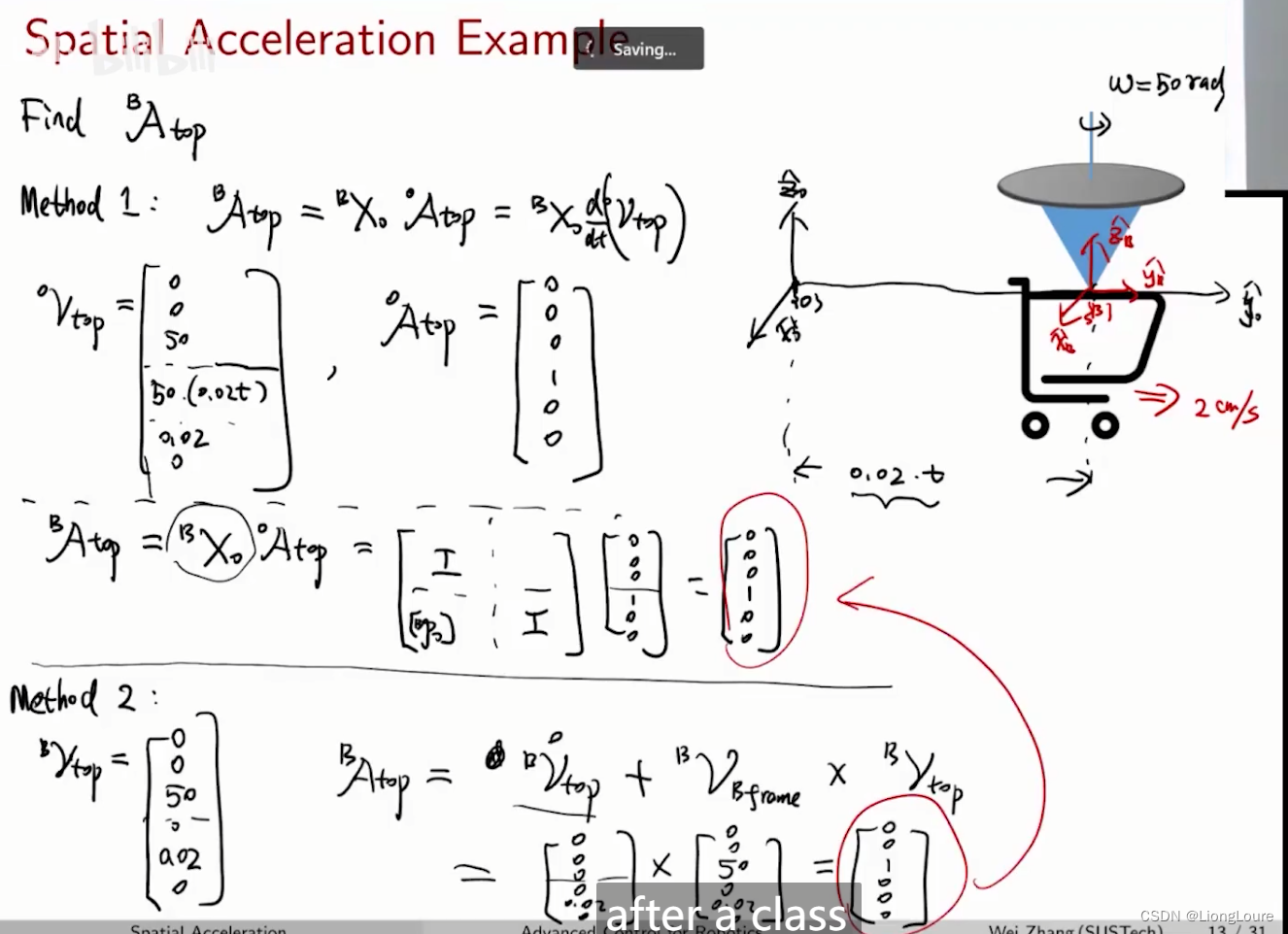
EXAMPLE:

2. Spatial Force(Wrench)

Consider a rigid body with many forces on it and fix an arbitrary point $O$ in space

The net effect of these forces can be expressed as:

• A force $f$ , acting along a line passing through $O$ —— $\vec{f}=\sum {{\vec{f}}_{\mathrm{i}}}_{\mathrm{i}}$
• A moment ${\vec{m}}_{\mathrm{O}}$ about point $O$ —— ${\vec{m}}_{\mathrm{O}}=\sum {\vec{R}}_{\mathrm{Pi}}^O\times {\vec{f}}_{\mathrm{i}}$

Spatial Force(Wrench) : is given by the 6D vector
$$\mathcal{F}=\left[\begin{array}{c}{\vec{m}}_{\mathrm{O}}\\ \vec{f}\end{array}\right]$$

What is we choose reference point to $Q$?
${\vec{m}}_{\mathrm{Q}}=\sum {\vec{R}}_{\mathrm{Pi}}^Q\times {\vec{f}}_{\mathrm{i}}=\sum \left({\vec{R}}_{\mathrm{O}}^Q+{\vec{R}}_{\mathrm{Pi}}^O\right)\times {\vec{f}}_{\mathrm{i}}={\vec{m}}_{\mathrm{O}}+\sum {\vec{R}}_{\mathrm{O}}^Q\times {\vec{f}}_{\mathrm{i}}$

2.1 Spatial Force in Pluecker Coordinate Systems

Given a frame { A } \left\{ A \right\} {A}, the Plueker coordinate of a spatial force $\mathcal{F}$ is given by ${\mathcal{F}}^A=\left[\begin{array}{c}{\vec{m}}_{\mathrm{O}}^A\\ {\vec{f}}^A\end{array}\right]$

Coordinate transform :
$$\{\begin{array}{l}{\vec{f}}^A=\left[Q_{\mathrm{B}}^A\right]{\vec{f}}^B\\ {\vec{m}}_{\mathrm{O}}^A=\left[Q_{\mathrm{B}}^A\right]{\vec{m}}_{\mathrm{O}}^B+{\vec{R}}_{\mathrm{B}}^A\times \left[Q_{\mathrm{B}}^A\right]{\vec{f}}^B\end{array}?{\mathcal{F}}^A={\left[X_{\mathrm{B}}^A\right]}^{\mathrm{T}}{\mathcal{F}}^B={\left[X_{\mathrm{B}}^A\right]}^{?}{\mathcal{F}}^B$$

2.2 Wrench-Twist Pair and Power

Recall that for a point mass with linear velocity $\vec{v}$ and a linear force $\vec{f}$ . Then we know that the power (instantaneous work done by $\vec{f}$ ) is given by : $\vec{f}?\vec{v}={\vec{f}}^{\mathrm{T}}\vec{v}$

This relation can be generalized to spatial force (i.e. wrench) and spatial velocity (i.e. twist)

Suppose a rigid body has a twist ${\mathcal{V}}^A=\left({\vec{\omega }}^A,{\vec{v}}_{\mathrm{O}}^A\right)$ and a wrench ${\mathcal{F}}^A=\left({\vec{m}}_{\mathrm{O}}^A,{\vec{f}}^A\right)$ acts on the body. Then the power is simply
$$P={\left({\mathcal{V}}^A\right)}^{\mathrm{T}}{\mathcal{F}}^A={\left({\mathcal{F}}^A\right)}^{\mathrm{T}}{\mathcal{V}}^A={\left({\vec{\omega }}^A\right)}^{\mathrm{T}}{\vec{m}}_{\mathrm{O}}^A+{\left({\vec{v}}_{\mathrm{O}}^A\right)}^{\mathrm{T}}{\vec{f}}^A$$

2.3 Joint Torque

Consider a link attached to a 1-dof joint(r.g. revolute or prismatic). be the screw axis of the joint. Then the power produced by the joint is $\mathcal{V}=\hat{\mathcal{S}}\dot{\theta }$

$\mathcal{F}$ be the wrench provided by the joint. Then the power produced by the joint is $$P={\left(\mathcal{V}\right)}^{\mathrm{T}}\mathcal{F}={\left(\hat{\mathcal{S}}\dot{\theta }\right)}^{\mathrm{T}}\mathcal{F}=\left({\hat{\mathcal{S}}}^{\mathrm{T}}\mathcal{F}\right)\dot{\theta }=\tau \dot{\theta }$$

$\tau ={\hat{\mathcal{S}}}^{\mathrm{T}}\mathcal{F}={\mathcal{F}}^{\mathrm{T}}\hat{\mathcal{S}}$ is the projection of the wrench onto the screw axis, i.e. the effective part of the wrench

Often times, $\tau$ is referred to as joint “torque” or generalized force

3. Spatial Momentum

笔者待整理： 链接

3.1 Rotational Interial

• Recall momentum for point mass:

笔者待整理： 链接

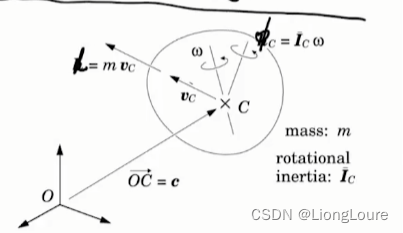
$$\mathcal{H}=\left[\begin{array}{c}\vec{h}\\ \vec{p}\end{array}\right]\in {\mathbb{R}}^6$$

3.2 Change Reference for Momentum

• Spatial momentum transforms in the same way as spatial forces:
  $${\mathcal{H}}^A={\left[X_{\mathrm{C}}^A\right]}^{?}{\mathcal{H}}^C$$
  ${\mathcal{H}}^C=\left[\begin{array}{c}{\vec{h}}_{\mathrm{Body}/\mathrm{C}}^C\\ {\vec{p}}^C\end{array}\right],{\mathcal{H}}^A=\left[\begin{array}{c}{\vec{h}}_{\mathrm{A}}^A\\ {\vec{p}}^A\end{array}\right]=\left[\begin{array}{c}\left[Q_{\mathrm{C}}^A\right]{\vec{h}}_{\mathrm{Body}/\mathrm{C}}^C?{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{C}}^A\left[Q_{\mathrm{C}}^A\right]{\vec{p}}^C\\ \left[Q_{\mathrm{C}}^A\right]{\vec{p}}^C\end{array}\right]=\left[\begin{array}{cc}\left[Q_{\mathrm{C}}^A\right]& ?{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{C}}^A\left[Q_{\mathrm{C}}^A\right]\\ 0& \left[Q_{\mathrm{C}}^A\right]\end{array}\right]\left[\begin{array}{c}{\vec{h}}_{\mathrm{Body}/\mathrm{C}}^C\\ {\vec{p}}^C\end{array}\right]={\left[X_{\mathrm{C}}^A\right]}^{?}\left[\begin{array}{c}{\vec{h}}_{\mathrm{Body}/\mathrm{C}}^C\\ {\vec{p}}^C\end{array}\right]$

3.3 Spatial Inertia

Inertia of a rigid body defines linear relationship between velocity and momentum

Spacial inertia $\mathcal{I}$ is the one such that
$$\mathcal{H}=\mathcal{IV}$$
Let { M } \left\{ M \right\} {M} be a frame whose origin coincide with CoM. Then
$${\mathcal{I}}_{\mathrm{Body}/\mathrm{CoM}}^M=\left[\begin{array}{cc}{I}_{\mathrm{Body}/\mathrm{CoM}}^M& 0\\ 0& m_{\mathrm{total}}{E}_{3\times 3}\end{array}\right]G$$

• Spatial inertia wrt another frame { F } \left\{ F \right\} {F}:
  $${\mathcal{I}}^F={\left[X_{\mathrm{M}}^F\right]}^{?}{\mathcal{I}}^M\left[X_{\mathrm{F}}^M\right]$$

Special case : $\left[Q_{\mathrm{F}}^M\right]=E_{3\times 3}$
$\left[X_{\mathrm{M}}^F\right]=\left[\begin{array}{cc}{E}_{3\times 3}& 0\\ {\widetilde{\stackrel{⃗}{R}}}_{\mathrm{M}}^F& E_{3\times 3}\end{array}\right]?{\mathcal{I}}^F=\left[\begin{array}{cc}{\mathcal{I}}^M+m_{\mathrm{total}}{{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{M}}^F}^{\mathrm{T}}{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{M}}^F& m_{\mathrm{total}}{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{M}}^F\\ m_{\mathrm{total}}{\widetilde{\stackrel{⃗}{R}}}_{\mathrm{M}}^F& m_{\mathrm{total}}{E}_{3\times 3}\end{array}\right]$

4. Newton-Euler Equation using Spatial Vectors

4.1 Cross Product for Spatial Force and Momentum

Assume frame $A$ is moving with velocity ${\mathcal{V}}_{\mathrm{A}}^A$
$${\left(\frac{\mathrm{d}}{\mathrm{d}t}\mathcal{F}\right)}^A=\frac{\mathrm{d}}{\mathrm{d}t}{\mathcal{F}}^A+{\mathcal{V}}^A{\times }^{?}{\mathcal{F}}^A$$
$${\left(\frac{\mathrm{d}}{\mathrm{d}t}\mathcal{H}\right)}^A=\frac{\mathrm{d}}{\mathrm{d}t}{\mathcal{H}}^A+{\mathcal{V}}^A{\times }^{?}{\mathcal{H}}^A$$

where ${\times }^{?}$ defined as $\mathcal{V}=\left[\begin{array}{c}\vec{\omega }\\ \vec{v}\end{array}\right],\mathcal{F}=\left[\begin{array}{c}\vec{m}\\ \vec{f}\end{array}\right],\mathcal{V}{\times }^{?}\mathcal{F}=\left[\begin{array}{c}\widetilde{\stackrel{⃗}{\omega }}\vec{m}+\widetilde{\stackrel{⃗}{v}}\vec{f}\\ \widetilde{\stackrel{⃗}{\omega }}\vec{f}\end{array}\right]$, or equivately $\widetilde{\mathcal{V}{\times }^{?}}=\left[\begin{array}{cc}\widetilde{\stackrel{⃗}{\omega }}& \widetilde{\stackrel{⃗}{v}}\\ 0& \widetilde{\stackrel{⃗}{\omega }}\end{array}\right]$

Fact : $\widetilde{\mathcal{V}{\times }^{?}}={\tilde{\mathcal{V}}}^{\mathrm{T}}$

4.2 Newton-Euler Equation

• Newton-Euler equation :
  $$\mathcal{F}=\frac{\mathrm{d}}{\mathrm{d}t}\mathcal{H}=\mathcal{I}\mathcal{A}+{\tilde{\mathcal{V}}}^{\mathrm{T}}\mathcal{IV}$$
  (due to velocity is changing and account for the face that inertia is moving)

Adopting spatial vectors, the Newton-Euler equation has the same form in any frame

4.3 Derivations of Newton-Euler Equation

$$\begin{gathered} \frac{\mathrm{d}}{\mathrm{d}t}{\mathcal{H}}^O=\frac{\mathrm{d}}{\mathrm{d}t}\left({\mathcal{I}}^O{\mathcal{V}}^O\right)={\dot{\mathcal{I}}}^O{\mathcal{V}}^O+{\mathcal{I}}^O{\mathcal{A}}^O=\frac{\mathrm{d}}{\mathrm{d}t}\left({\left[X_{\mathrm{B}}^O\right]}^{?}{\mathcal{I}}^B\left[X_{\mathrm{O}}^B\right]\right){\mathcal{V}}^O+{\mathcal{I}}^O{\mathcal{A}}^O \\ ={\left[{\dot{X}}_{\mathrm{B}}^O\right]}^{?}{\mathcal{I}}^B\left[X_{\mathrm{O}}^B\right]{\mathcal{V}}^O+{\left[X_{\mathrm{B}}^O\right]}^{?}{\mathcal{I}}^B\left[{\dot{X}}_{\mathrm{O}}^B\right]{\mathcal{V}}^O+{\mathcal{I}}^O{\mathcal{A}}^O \\ ={{\tilde{\mathcal{V}}}_{\mathrm{B}}^O}^{\mathrm{T}}{\left[X_{\mathrm{B}}^O\right]}^{?}{\mathcal{I}}^B\left[X_{\mathrm{O}}^B\right]{\mathcal{V}}^O?{\left[X_{\mathrm{B}}^O\right]}^{?}{\mathcal{I}}^B\left[X_{\mathrm{O}}^B\right]{{\tilde{\mathcal{V}}}_{\mathrm{B}}^O}^{\mathrm{T}}{{\mathcal{V}}^O}_{\nearrow 0}+{\mathcal{I}}^O{\mathcal{A}}^O \\ ={{\tilde{\mathcal{V}}}_{\mathrm{B}}^O}^{\mathrm{T}}{\mathcal{I}}^O{\mathcal{V}}^O+{\mathcal{I}}^O{\mathcal{A}}^O \end{gathered}$$

Note :
$\{\begin{array}{l}\left[{\dot{X}}_{\mathrm{B}}^O\right]={\tilde{\mathcal{V}}}_{\mathrm{B}}^O\left[X_{\mathrm{B}}^O\right]\\ \left[X_{\mathrm{B}}^O\right]\left[X_{\mathrm{O}}^B\right]=E\end{array}?\left[{\dot{X}}_{\mathrm{B}}^O\right]\left[X_{\mathrm{O}}^B\right]+\left[X_{\mathrm{B}}^O\right]\left[{\dot{X}}_{\mathrm{O}}^B\right]=0?\left[{\dot{X}}_{\mathrm{O}}^B\right]=?\left[X_{\mathrm{O}}^B\right]\left[{\dot{X}}_{\mathrm{B}}^O\right]\left[X_{\mathrm{O}}^B\right]=?\left[X_{\mathrm{O}}^B\right]{\tilde{\mathcal{V}}}_{\mathrm{B}}^O$
Frame B is attached to the body , ${\mathcal{V}}_B={\mathcal{V}}_{Body},{\mathcal{I}}^B$ is constant