算法第十三天-组合总和Ⅱ

组合总和Ⅱ

题目要求

解题思路

按顺序搜索，设置合理的变量，在搜索的过程中判断是否会出现重复集结果。重点理解对输入数组排序的作用和参考代码中 大剪枝和小剪枝 的意思

这道题域上一问的区别在于：

• 第39题：candidates中的数字可以无限制重复被选取；
• 第40题：candidates中的每个数字在每个组合中只能使用一次；

相同点在于：相同数字列表的不同排列被视为一个结果。

如何去掉重复的集合（重点）
为了使得解集不包含重复的组合。可以从以下两种方案思考：

• 使用 hash 天然去重功能，但是编码相对复杂；
• 使用和第39题和第15题相似的思路：不重复就不需要按顺序搜索，**在搜索过程中检测分支是否会出现重复结果。**注意：在这里的顺序不仅仅指数组candidates有序，还指按照一定顺序搜索结果。

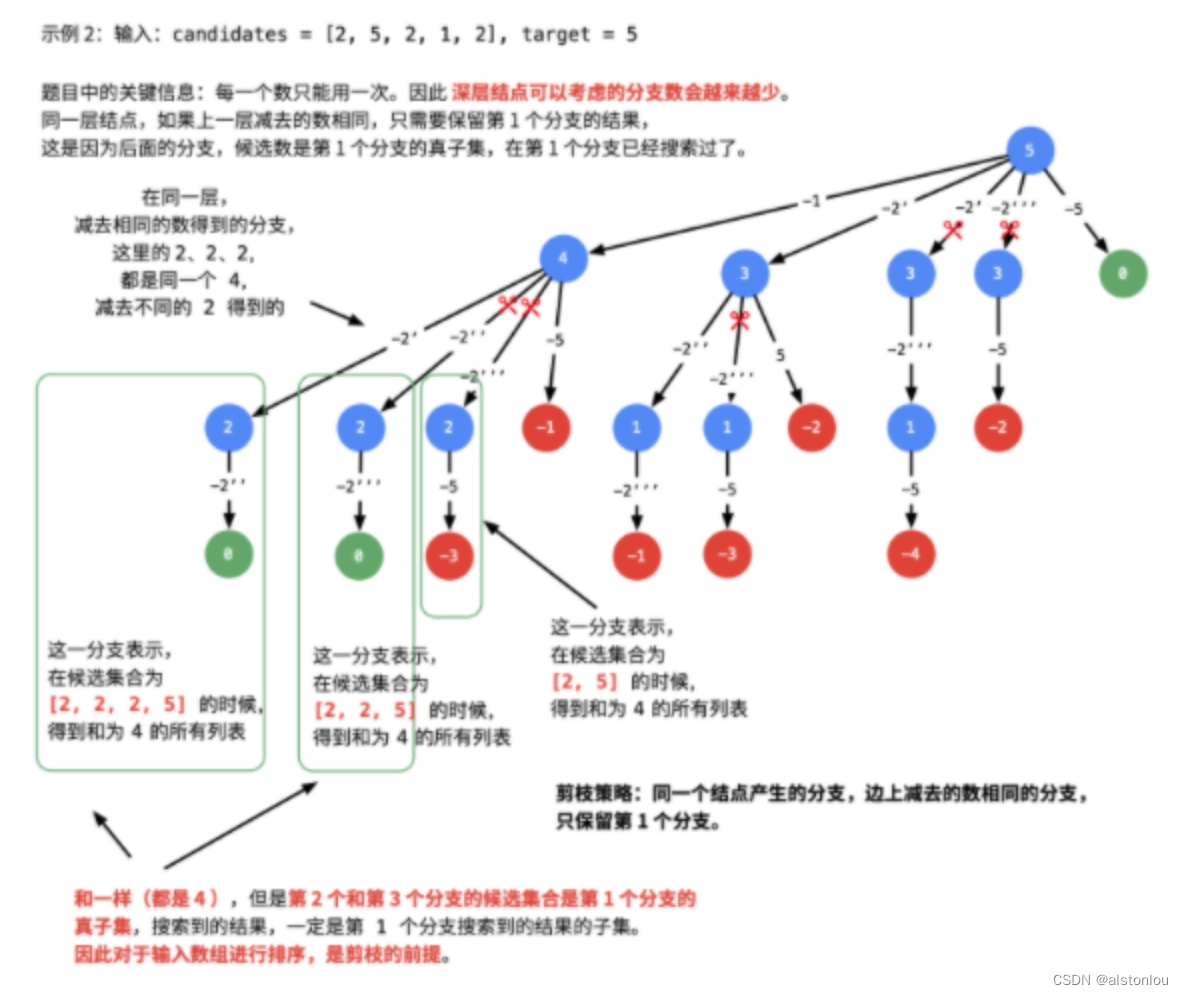
由39题我们知道，数组candidates有序，也是DFS 过程中实现[剪枝]的前提。
将数组先排序的思路来自于这个问题：去掉一个数组中重复的元素。很容易想到的方案是：先对数组升序排列，重复的元素一定不是排好序以后相同的连续数组区域的第1个元素。也就是说，剪枝发生在：**同一层数值相同的节点第2，3，…个节点，因为数值相同的第1个节点已经搜索出了包含了这个数值的全部结果，**同一层的其他节点，候选数的个数更少，搜索出的结果一定不会比第1个节点更多，并且是第一个节点的子集。

代码

from typing import List
class Solution:
    def combinationSum2(self, candidates: List[int], target: int) -> List[List[int]]:
        def dfs(begin, path, residue):
            if residue == 0:
                res.append(path[:])
                return
            for index in range(begin, size):
                if candidates[index] > residue:
                    break
                if index > begin and candidates[index - 1] == candidates[index]:
                    continue
                path.append(candidates[index])
                dfs(index + 1, path, residue - candidates[index])
                path.pop()
        size = len(candidates)
        if size == 0:
            return []
        candidates.sort()
        res = []
        dfs(0, [], target)
        return res

复杂度分析

时间复杂度：$O(2^n?n)$，其中n为candidates的长度。在大多数的递归+回溯的题目中我们无法给出一个严格的渐近界限，故这里只分析一个较为宽松的渐近上界。
空间复杂度：$O(n)$