POJ：2826

?题目链接：http://poj.org/problem?id=2826

?接不到水的情况：

1 两线段不相交
2 有一线段跟x平行
3 两线段平行或重合
4 有交点但交点是其中一个线段的上端点
5 有一块木板挡住了接水口,如图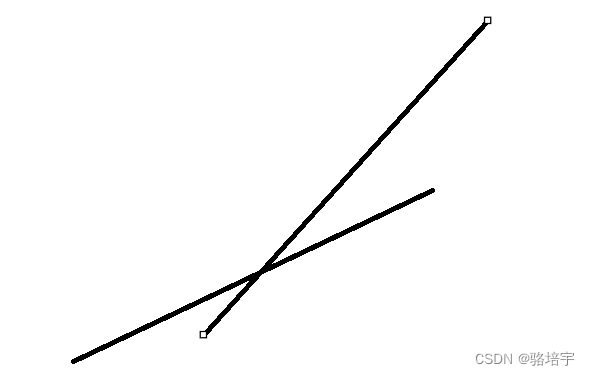

/*
floor();//向下取整  ceil();//向上取整  round();//四舍五入
判等时不要直接使用=
*/
#include  <map>
#include  <set>
#include  <cmath>
#include  <queue>
#include  <cstdio>
#include  <vector>
#include  <climits>
#include  <cstring>
#include  <cstdlib>
#include  <iostream>
#include  <algorithm>
using namespace std;
const double pi = acos(-1.0);
const double inf = 1e100;
/*
误差判断
*/
const double eps = 1e-9;
int dcmp(double x, double y){
    if(fabs(x - y) < eps)
        return 0;
    if(x > y)
        return 1;
    return -1;
}
int sgn(double d){
    if(fabs(d) < eps)
        return 0;
    if(d > 0)
        return 1;
    return -1;
}
/*---------------------------------------------------------------------------------------*/
//点
struct Point{
	double x, y,z;
    Point(double x = 0, double y = 0, double z = 0):x(x),y(y),z(z){}
};
//向量
typedef Point Vector;
//运算
Vector operator + (Vector A, Vector B){
    return Vector(A.x+B.x, A.y+B.y,A.z+B.z);
}
Vector operator - (Vector A, Vector B){
    return Vector(A.x-B.x, A.y-B.y,A.z-B.z);
}
Vector operator * (Vector A, double p){
    return Vector(A.x*p, A.y*p,A.z*p);
}
Vector operator / (Vector A, double p){
    return Vector(A.x/p, A.y/p,A.z/p);
}
bool operator == (Point A,Point B){
	return sgn(A.x-B.x)==0&&sgn(A.y-B.y)==0;
}
bool P_cmp1(Point a,Point b){
    if(a.x==b.x)
    	return a.y<b.y;
    return a.x<b.x;
}
//向量数量积  向量α在向量β的投影于向量β的长度乘积（带方向）
double Dot(Vector A, Vector B){
    return A.x*B.x + A.y*B.y + A.z*B.z;
}
//向量向量积  向量α与β所张成的平行四边形的有向面积
double Cross(Vector A, Vector B){
    return A.x*B.y-A.y*B.x;
}
//取模
double Length(Vector A){
    return sqrt(Dot(A, A));
}
//计算夹角
double Angle(Vector A, Vector B){
    return acos(Dot(A, B) / Length(A) / Length(B));
}
//向量旋转
Vector Rotate(Vector A, double rad){//rad为弧度 且为逆时针旋转的角
    return Vector(A.x*cos(rad)-A.y*sin(rad), A.x*sin(rad)+A.y*cos(rad));
}
Vector Normal(Vector A){//向量A左转90°的单位法向量
    double L = Length(A);
    return Vector(-A.y/L, A.x/L);
}
//三角形外接圆圆心
Point Excenter(Point a, Point b, Point c){
    double a1 = b.x - a.x;
    double b1 = b.y - a.y;
    double c1 = (a1*a1 + b1*b1)/2;
    double a2 = c.x - a.x;
    double b2 = c.y - a.y;
    double c2 = (a2*a2 + b2*b2)/2;
    double d = a1*b2 - a2*b1;
    return Point(a.x + (c1*b2 - c2*b1)/d, a.y + (a1*c2 - a2*c1)/d);
}
//两点距离
double dis(Point a,Point b){
	return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y)+(a.z-b.z)*(a.z-b.z));
}
/*------------------------------------------------------------------------------------------------*/
//线
struct Line{//直线定义
    Point v, p;
    //Line(Point v, Point p):v(v), p(p){}
    Point point(double t){//返回点P = v + (p - v)*t
        return v + (p - v)*t;
    }
};
//判断点是否在线上
bool PointOnline(Line A,Point a){
	Vector v1(A.p.x-a.x,A.p.y-a.y,A.p.z-a.z);
	Vector v2(A.v.x-a.x,A.v.y-a.y,A.v.z-a.z);
	return !(Cross(v1,v2));
}
//计算两直线交点 必须保证直线相交，否则将会出现除以零的情况
Point GetLineIntersection(Point a1, Point a2, Point b1, Point b2){
	Vector v = a1-a2;
	Vector w = b1-b2;
	Vector u = a1-b1;
    double t = Cross(w, u)/Cross(v, w);
    return a1+v*t;
}
//点P到直线AB距离公式
double DistanceToLine(Point P, Point A, Point B){
    Vector v1 = B-A, v2 = P-A;
    return fabs(Cross(v1, v2)/Length(v1));
}
//点P到线段AB距离公式
double DistanceToSegment(Point P, Point A, Point B){
    if(A.x == B.x&&A.y == B.y&&A.z == B.z)
        return Length(P-A);
    Vector v1 = B-A, v2 = P-A, v3 = P-B;
    if(dcmp(Dot(v1, v2),0) < 0)
        return Length(v2);
    if(dcmp(Dot(v1, v3),0) > 0)
        return Length(v3);
    return DistanceToLine(P, A, B);
}
//点P在直线AB上的投影点
Point GetLineProjection(Point P, Point A, Point B){
    Vector v = B-A;
    return A+v*(Dot(v, P-A)/Dot(v, v));
}
//判断点是否在线段端上
bool OnSegment(Point p, Point a1, Point a2){
    return dcmp(Cross(a1-p, a2-p),0) == 0 && dcmp(Dot(a1-p, a2-p),0) <= 0;
}
//判断线段相交
bool SegmentProperIntersection(Point a1, Point a2, Point b1, Point b2){
    double c1 = Cross(a2-a1, b1-a1), c2 = Cross(a2-a1, b2-a1);
    double c3 = Cross(b2-b1, a1-b1), c4 = Cross(b2-b1, a2-b1);
    //if判断控制是否允许线段在端点处相交，根据需要添加
    if(!sgn(c1) || !sgn(c2) || !sgn(c3) || !sgn(c4)){
        bool f1 = OnSegment(b1, a1, a2);
        bool f2 = OnSegment(b2, a1, a2);
        bool f3 = OnSegment(a1, b1, b2);
        bool f4 = OnSegment(a2, b1, b2);
        bool f = (f1|f2|f3|f4);
        return f;
    }
    return (sgn(c1)*sgn(c2) < 0 && sgn(c3)*sgn(c4) < 0);
}
//点c是否在线段ab的左侧 左1 线上0 右-1
int ToLeftTest(Point a, Point b, Point c){
    if( Cross(b - a, c - b) > 0) return 1;
    else if( Cross(b - a, c - b) == 0) return 0;
    else return -1;
}
/*------------------------------------------------------------------------*/
//多边形
//求凸包 多边形p  凸包ch
int ConvexHull(Point *p,int n,Point *ch){
	n=unique(p,p+n)-p;//去重
	sort(p,p+n,P_cmp1);
	int v=0;
	for(int i=0;i<n;i++){
		while(v>1&&Cross(ch[v-1]-ch[v-2],p[i]-ch[v-1])<=0)
			v--;
		ch[v++]=p[i];
	}
	int j=v;
	for(int i=n-2;i>=0;i--){
		while(v>j&&Cross(ch[v-1]-ch[v-2],p[i]-ch[v-1])<=0)
			v--;
		ch[v++]=p[i];
	}
	if(n>1) v--;
	return v;
}
//多边形有向面积
double PolygonArea(Point* p, int n){//p为端点集合，n为端点个数
    double s = 0;
    for(int i = 1; i < n-1; ++i)
        s += Cross(p[i]-p[0], p[i+1]-p[0]);
    return s;
}
//判断点是否在多边形内，若点在多边形内返回1，在多边形外部返回0，在多边形上返回-1
int isPointInPolygon(Point p, vector<Point> poly){
    int wn = 0;
    int n = poly.size();
    for(int i = 0; i < n; ++i){
        if(OnSegment(p, poly[i], poly[(i+1)%n])) return -1;
        int k = sgn(Cross(poly[(i+1)%n] - poly[i], p - poly[i]));
        int d1 = sgn(poly[i].y - p.y);
        int d2 = sgn(poly[(i+1)%n].y - p.y);
        if(k > 0 && d1 <= 0 && d2 > 0) wn++;
        if(k < 0 && d2 <= 0 && d1 > 0) wn--;
    }
    if(wn != 0)
        return 1;
    return 0;
}
/************************************************************************************/
bool cmp(Point a,Point b)
{
	return (a.y>b.y);
}
void isok()
{
	Point a,b,c,d;
	cin>>a.x>>a.y>>b.x>>b.y>>c.x>>c.y>>d.x>>d.y;
	//保证a和c为上端点且ba的角度小于dc
	if(a.y<b.y)swap(a,b);
	if(c.y<d.y)swap(c,d);
	Vector X(1,0);
	if(Angle(X,a-b)>Angle(X,c-d))swap(a,c),swap(b,d);
	//两线段不相交
	if(!SegmentProperIntersection(a,b,c,d))
	{
		printf("0.00\n");
		return ;
	}
	//有一个于x平行
	if(a.y==b.y||c.y==d.y)
	{
		printf("0.00\n");
		return ;
	}
	//两线段平行或重合
	Vector u=a-b,w=c-d;
	if(u.x*w.y==u.y*w.x)
	{
		printf("0.00\n");
		return ;
	}
	//得到三角形三点g,h,j
	Point g,h,j;
	g=GetLineIntersection(a,b,c,d);
	if(g==a||g==c)//相交点在高处的端点
	{
		printf("0.00\n");
		return ;
	}
	//有长板挡住接水口
	if(a.x>g.x&&c.x>g.x&&a.x<=c.x)
	{
		printf("0.00\n");
		return ;
	}
	if(a.x<g.x&&c.x<g.x&&a.x<=c.x)
	{
		printf("0.00\n");
		return ;
	}
	if(a.y<c.y)
	{
		h=a;
		Point x(10003,h.y);
		j=GetLineIntersection(c,d,x,h);
	}
	else if(a.y>c.y)
	{
		h=c;
		Point x(10003,h.y);
		j=GetLineIntersection(a,b,x,h);
	}
	else
		h=a,j=c;
	double ans=(j.y-g.y)*(fabs(j.x-h.x))/2.0;
	printf("%.2lf\n",ans);
}
int main()
{
	int t;
	cin>>t;
	while(t--)
		isok();
}