【LeetCode题目详解】59. 螺旋矩阵 II 54. 螺旋矩阵 LCR 146. 螺旋遍历二维数组（c++）

这篇文章的题目稍微难一点

题目建议：??本题关键还是在转圈的逻辑，在二分搜索中提到的区间定义，在这里又用上了。?

一、59. 螺旋矩阵 II

题目：

给你一个正整数?n ，生成一个包含 1 到?n2?所有元素，且元素按顺时针顺序螺旋排列的?n x n 正方形矩阵 matrix 。

示例 1：

输入：n = 3
输出：[[1,2,3],[8,9,4],[7,6,5]]

示例 2：

输入：n = 1
输出：[[1]]

提示：

• 1 <= n <= 20

思路

这道题目可以说在面试中出现频率较高的题目，本题并不涉及到什么算法，就是模拟过程，但却十分考察对代码的掌控能力。

要如何画出这个螺旋排列的正方形矩阵呢？

相信很多同学刚开始做这种题目的时候，上来就是一波判断猛如虎。

结果运行的时候各种问题，然后开始各种修修补补，最后发现改了这里那里有问题，改了那里这里又跑不起来了。

大家还记得我们在这篇文章数组：每次遇到二分法，都是一看就会，一写就废

(opens new window)中讲解了二分法，提到如果要写出正确的二分法一定要坚持循环不变量原则。

而求解本题依然是要坚持循环不变量原则。

模拟顺时针画矩阵的过程:

• 填充上行从左到右
• 填充右列从上到下
• 填充下行从右到左
• 填充左列从下到上

由外向内一圈一圈这么画下去。

可以发现这里的边界条件非常多，在一个循环中，如此多的边界条件，如果不按照固定规则来遍历，那就是一进循环深似海，从此offer是路人。

这里一圈下来，我们要画每四条边，这四条边怎么画，每画一条边都要坚持一致的左闭右开，或者左开右闭的原则，这样这一圈才能按照统一的规则画下来。

那么我按照左闭右开的原则，来画一圈，大家看一下：

这里每一种颜色，代表一条边，我们遍历的长度，可以看出每一个拐角处的处理规则，拐角处让给新的一条边来继续画。

这也是坚持了每条边左闭右开的原则。

一些同学做这道题目之所以一直写不好，代码越写越乱。

就是因为在画每一条边的时候，一会左开右闭，一会左闭右闭，一会又来左闭右开，岂能不乱。

代码如下，已经详细注释了每一步的目的，可以看出while循环里判断的情况是很多的，代码里处理的原则也是统一的左闭右开。

整体C++代码如下：

class Solution {
public:
    vector<vector<int>> generateMatrix(int n) {
        vector<vector<int>> res(n, vector<int>(n, 0)); // 使用vector定义一个二维数组
        int startx = 0, starty = 0; // 定义每循环一个圈的起始位置
        int loop = n / 2; // 每个圈循环几次，例如n为奇数3，那么loop = 1 只是循环一圈，矩阵中间的值需要单独处理
        int mid = n / 2; // 矩阵中间的位置，例如：n为3， 中间的位置就是(1，1)，n为5，中间位置为(2, 2)
        int count = 1; // 用来给矩阵中每一个空格赋值
        int offset = 1; // 需要控制每一条边遍历的长度，每次循环右边界收缩一位
        int i,j;
        while (loop --) {
            i = startx;
            j = starty;

            // 下面开始的四个for就是模拟转了一圈
            // 模拟填充上行从左到右(左闭右开)
            for (j = starty; j < n - offset; j++) {
                res[startx][j] = count++;
            }
            // 模拟填充右列从上到下(左闭右开)
            for (i = startx; i < n - offset; i++) {
                res[i][j] = count++;
            }
            // 模拟填充下行从右到左(左闭右开)
            for (; j > starty; j--) {
                res[i][j] = count++;
            }
            // 模拟填充左列从下到上(左闭右开)
            for (; i > startx; i--) {
                res[i][j] = count++;
            }

            // 第二圈开始的时候，起始位置要各自加1， 例如：第一圈起始位置是(0, 0)，第二圈起始位置是(1, 1)
            startx++;
            starty++;

            // offset 控制每一圈里每一条边遍历的长度
            offset += 1;
        }

        // 如果n为奇数的话，需要单独给矩阵最中间的位置赋值
        if (n % 2) {
            res[mid][mid] = count;
        }
        return res;
    }
};

• 时间复杂度 O(n^2): 模拟遍历二维矩阵的时间
• 空间复杂度 O(1)

• 类似题目

• 54.螺旋矩阵
• (opens new window)
• 剑指Offer 29.顺时针打印矩阵
• (opens new window)

二、54. 螺旋矩阵

题目：

给你一个 m 行 n 列的矩阵?matrix ，请按照 顺时针螺旋顺序 ，返回矩阵中的所有元素。

示例 1：

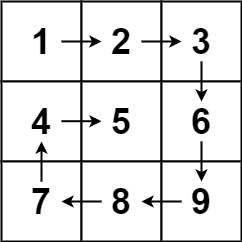
输入：matrix = [[1,2,3],[4,5,6],[7,8,9]]
输出：[1,2,3,6,9,8,7,4,5]

示例 2：

输入：matrix = [[1,2,3,4],[5,6,7,8],[9,10,11,12]]
输出：[1,2,3,4,8,12,11,10,9,5,6,7]

提示：

• m == matrix.length
• n == matrix[i].length
• 1 <= m, n <= 10
• -100 <= matrix[i][j] <= 100

题解：

两种题解：一种是根据上面那一题的思路去改的，稍微复杂一点

class Solution {
public:
    vector<int> spiralOrder(vector<vector<int>>& matrix) {
        if (matrix.size() == 0 || matrix[0].size() == 0) {
            return {};
        }
        vector<int> res;
        int i, j;
        int m = matrix.size(), n = matrix[0].size();
        int startx = 0, starty = 0;
        int offset = 1;

        // 特判：只有一行
        if (m == 1) {
            return matrix[0];
        }
        // 特判：只有一列
        if (n == 1) {
            for (i = 0; i < m; i++) {
                res.push_back(matrix[i][0]);
            }
            return res;
        }

        while (startx <= n - offset && starty <= m - offset) {
            i = startx;
            j = starty;

            for (j = starty; j < n - offset && res.size() < m * n; j++) {
                res.push_back(matrix[i][j]);
            }
            for (i = startx; i < m - offset && res.size() < m * n; i++) {
                res.push_back(matrix[i][j]);
            }
            for (; j > starty && res.size() < m * n; j--) {
                res.push_back(matrix[i][j]);
            }
            for (; i > startx && res.size() < m * n; i--) {
                res.push_back(matrix[i][j]);
            }

            startx++;
            starty++;

            offset += 1;
        }

        if (res.size() < m * n) {
            if (n % 2 && m % 2) {
                res.push_back(matrix[i][j]);
            } else if (n % 2) {
                for (; i >= startx && res.size() < m * n; i--) {
                    res.push_back(matrix[i][j]);
                }
            } else if (m % 2) {
                for (; j >= starty && res.size() < m * n; j--) {
                    res.push_back(matrix[i][j]);
                }
            }
        }

        return res;
    }
};

第二种是力扣上的题解，比较简洁一点

可以将矩阵看成若干层，首先输出最外层的元素，其次输出次外层的元素，直到输出最内层的元素。

定义矩阵的第 kkk 层是到最近边界距离为 kkk 的所有顶点。例如，下图矩阵最外层元素都是第 111 层，次外层元素都是第 222 层，剩下的元素都是第 333 层。

[[1, 1, 1, 1, 1, 1, 1],
?[1, 2, 2, 2, 2, 2, 1],
?[1, 2, 3, 3, 3, 2, 1],
?[1, 2, 2, 2, 2, 2, 1],
?[1, 1, 1, 1, 1, 1, 1]]

对于每层，从左上方开始以顺时针的顺序遍历所有元素。假设当前层的左上角位于 (top,left)(\textit{top}, \textit{left})(top,left)，右下角位于 (bottom,right)(\textit{bottom}, \textit{right})(bottom,right)，按照如下顺序遍历当前层的元素。

??? 从左到右遍历上侧元素，依次为 (top,left)(\textit{top}, \textit{left})(top,left) 到 (top,right)(\textit{top}, \textit{right})(top,right)。

??? 从上到下遍历右侧元素，依次为 (top+1,right)(\textit{top} + 1, \textit{right})(top+1,right) 到 (bottom,right)(\textit{bottom}, \textit{right})(bottom,right)。

??? 如果 left<right\textit{left} < \textit{right}left<right 且 top<bottom\textit{top} < \textit{bottom}top<bottom，则从右到左遍历下侧元素，依次为 (bottom,right?1)(\textit{bottom}, \textit{right} - 1)(bottom,right?1) 到 (bottom,left+1)(\textit{bottom}, \textit{left} + 1)(bottom,left+1)，以及从下到上遍历左侧元素，依次为 (bottom,left)(\textit{bottom}, \textit{left})(bottom,left) 到 (top+1,left)(\textit{top} + 1, \textit{left})(top+1,left)。

遍历完当前层的元素之后，将 left\textit{left}left 和 top\textit{top}top 分别增加 111，将 right\textit{right}right 和 bottom\textit{bottom}bottom 分别减少 111，进入下一层继续遍历，直到遍历完所有元素为止。

class Solution {
public:
    vector<int> spiralOrder(vector<vector<int>>& matrix) {
        if (matrix.size() == 0 || matrix[0].size() == 0) {
            return {};
        }

        int rows = matrix.size(), columns = matrix[0].size();
        vector<int> order;
        int left = 0, right = columns - 1, top = 0, bottom = rows - 1;
        while (left <= right && top <= bottom) {
            for (int column = left; column <= right; column++) {
                order.push_back(matrix[top][column]);
            }
            for (int row = top + 1; row <= bottom; row++) {
                order.push_back(matrix[row][right]);
            }
            if (left < right && top < bottom) {
                for (int column = right - 1; column > left; column--) {
                    order.push_back(matrix[bottom][column]);
                }
                for (int row = bottom; row > top; row--) {
                    order.push_back(matrix[row][left]);
                }
            }
            left++;
            right--;
            top++;
            bottom--;
        }
        return order;
    }
};

复杂度分析

??? 时间复杂度：O(mn)O(mn)O(mn)，其中 mmm 和 nnn 分别是输入矩阵的行数和列数。矩阵中的每个元素都要被访问一次。

??? 空间复杂度：O(1)O(1)O(1)。除了输出数组以外，空间复杂度是常数。

三、LCR 146. 螺旋遍历二维数组

题目：

给定一个二维数组 array，请返回「螺旋遍历」该数组的结果。

螺旋遍历：从左上角开始，按照 向右、向下、向左、向上 的顺序 依次 提取元素，然后再进入内部一层重复相同的步骤，直到提取完所有元素。

示例 1：

输入：array = [[1,2,3],[8,9,4],[7,6,5]]
输出：[1,2,3,4,5,6,7,8,9]

示例 2：

输入：array ?= [[1,2,3,4],[12,13,14,5],[11,16,15,6],[10,9,8,7]]
输出：[1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16]

限制：

• 0 <= array.length <= 100
• 0 <= array[i].length <= 100

注意：本题与主站 54 题相同：. - 力扣（LeetCode）

题解：

这一题基本和上面那一题一样

class Solution {
    public int[] spiralArray(int[][] array) {
        if (array == null || array.length == 0 || array[0].length == 0) {
            return new int[0];
        }
        int rows = array.length, columns = array[0].length;
        int[] order = new int[rows * columns];
        int index = 0;
        int left = 0, right = columns - 1, top = 0, bottom = rows - 1;
        while (left <= right && top <= bottom) {
            for (int column = left; column <= right; column++) {
                order[index++] = array[top][column];
            }
            for (int row = top + 1; row <= bottom; row++) {
                order[index++] = array[row][right];
            }
            if (left < right && top < bottom) {
                for (int column = right - 1; column > left; column--) {
                    order[index++] = array[bottom][column];
                }
                for (int row = bottom; row > top; row--) {
                    order[index++] = array[row][left];
                }
            }
            left++;
            right--;
            top++;
            bottom--;
        }
        return order;
    }
}