智能机器人与旋量代数(9)

发布时间：2024年01月23日

Chapt 3. 螺旋运动与旋量代数

3.1 螺旋运动

螺旋运动是关于一条空间直线的一个旋转运动，并伴随沿此直线的一个平移。是一种刚体绕空间轴 s s s旋转 θ \theta θ角，再沿该轴平移距离 d d d的复合运动，类似螺母沿螺纹做进给运动的情形。
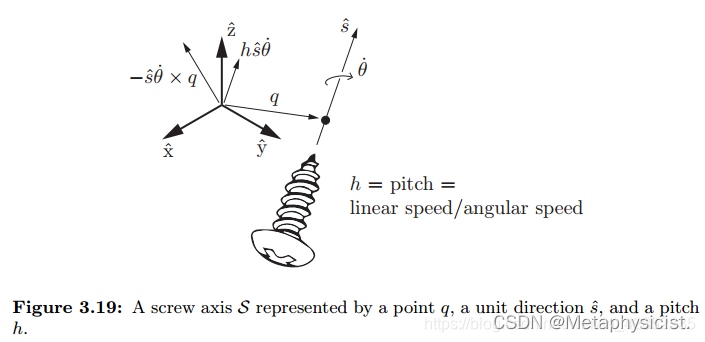
在这里插入图片描述

一些定义：

当 θ ≠ 0 \theta\neq0 θ=0 时，将移动量与转动量的比值 h = d θ h=\frac{d}{\theta} h=θd? 定义为螺旋的节距（或螺距）。因此，旋转 θ \theta θ 角后的纯移动量为 h θ h\theta hθ。

当 h = 0 h=0 h=0 时为纯转动；当 h = ∞ ( θ = 0 ) h=\infin (\theta=0) h=∞(θ=0) 时为纯移动。

定义3.1 螺旋运动的三要素是：轴线 s s s、螺距 h h h和转角 ρ \rho ρ。螺旋运动表示绕轴 s s s旋转 ρ = θ \rho=\theta ρ=θ，再沿该轴平移距离 h θ h\theta hθ的合成运动。如果 h = ∞ h=\infin h=∞，那么相应的螺旋运动即为沿轴 s s s移动距离 ρ \rho ρ的移动，记作 S ( s , h , ρ ) S(s,h,\rho) S(s,h,ρ).
p ( θ , h ) = r + R ( θ , s ) ( p ? r ) + h θ s , s ≠ 0 p(\theta,h)=r+R(\theta,s)(p-r)+h\theta s ,s\neq 0 p(θ,h)=r+R(θ,s)(p?r)+hθs,s=0
其中 R ( θ , s ) ∈ S O ( 3 ) R(\theta,s)\in SO(3) R(θ,s)∈SO(3)是关于空间某一条直线 s s s的刚体旋转运动。
表示成齐次坐标的形式为：
g ( p 1 ) = [ R ( I ? R ) r + h θ s 0 1 ] ( p 1 ) g\begin{pmatrix} p\\ 1 \end{pmatrix}=\begin{bmatrix} R & (I-R)r+h\theta s \\ 0 & 1 \end{bmatrix}\begin{pmatrix} p\\ 1 \end{pmatrix} g(p1?)=[R0?(I?R)r+hθs1?](p1?)
由此可得，刚体螺旋运动的描述为：
[ R ( I ? R ) r + h θ s 0 1 ] , s ≠ 0 \begin{bmatrix} R & (I-R)r+h\theta s \\ 0 & 1 \end{bmatrix},s\neq0 [R0?(I?R)r+hθs1?],s=0
Chasles-Mozzi定理(1830)：任意刚体运动都可以通过螺旋运动即通过绕某轴的转动与沿该轴移动的负荷运动实现。即：刚体运动与螺旋运动等价，螺旋运动是刚体运动，刚体运动也是螺旋运动。螺旋运动的无限小量为运动旋量。

Chasles理论推广：对于任意的刚体运动总能将其表达为螺旋运动，满足如下关系式：
[ R P 0 1 ] = [ R ( θ , s ) h θ s + ( I 3 ? R ) r 0 1 ] \begin{bmatrix} R & P\\ 0 & 1 \end{bmatrix}=\begin{bmatrix} R(\theta,s) &h \theta s+(I_3-R)r \\ 0 & 1 \end{bmatrix} [R0?P1?]=[R(θ,s)0?hθs+(I3??R)r1?]
更进一步，对于转动情况，定义矩阵指数与矩阵对数：

e x p : [ S ] θ ∈ s e ( 3 ) → T ∈ S E ( 3 ) . exp: [S]\theta\in se(3) \rightarrow T\in SE(3). exp:[S]θ∈se(3)→T∈SE(3).
l o g : T ∈ S E ( 3 ) → [ S ] θ ∈ s e ( 3 ) . log: T\in SE(3) \rightarrow [S]\theta\in se(3). log:T∈SE(3)→[S]θ∈se(3).

由Euler-Rodrigues formula 得如下命题：

令 S = ( ω , v ) S=(\omega,v) S=(ω,v)为螺旋轴，若 ∣ ∣ ω ∣ ∣ = 1 ||\omega||=1 ∣∣ω∣∣=1，则对于任意沿螺旋轴的距离 θ ∈ R \theta \in R θ∈R，都有
s [ S ] θ = [ e [ ω ] θ ( I θ + ( 1 ? c o s θ ) [ ω ] + ( θ ? s i n θ ) [ ω ] 2 ) v 0 1 ] s^{[S]\theta}=\begin{bmatrix} e^{[\omega]\theta} & (I\theta+(1-cos\theta)[\omega]+(\theta-sin\theta)[\omega]^2)v \\ 0 & 1 \end{bmatrix} s[S]θ=[e[ω]θ0?(Iθ+(1?cosθ)[ω]+(θ?sinθ)[ω]2)v1?]

Reference

[1] Lynch, K. M., & Park, F. C. (2017). Modern robotics. Cambridge University Press.

[2] Dai, J. S. (2014). Geometrical foundations and screw algebra for mechanisms and robotics. Higher Education Press, also Screw Algebra and Kinematic Approaches for Mechanisms and Robotics.

[3] Ding, X. L. (2021). Modern Mathematical Theory Foundation of Robotics. Beijing: Science Press.

[4] Gao, X. et al. (2017). Visual SLAM fourteen lessons: From theory to practice. Electronic Industry Press.

文章来源:https://blog.csdn.net/Metaphysicist/article/details/135701035
本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。
最新文章