秋招复习之数组与链表

发布时间：2024年01月04日

目录

前言

1 数组

初始化数组：

访问元素

插入元素

删除元素?

遍历数组：

查找元素?

扩容数组：

数组的优点与局限性

2 链表

初始化链表

插入节点

删除节点

访问节点

查找节点

数组 vs. 链表

常见链表类型

3 ?列表

初始化列表

访问元素

插入与删除元素

遍历列表

拼接列表

排序列表

列表实现

4 ? 内存与缓存

总结

前言

复习数组和链表，包括数组链表和列表，以及他们的一些基本操作，这里主要写的Java和cpp语言。

1 数组

初始化数组：

两种方式

Java

/* 初始化数组 */
int[] arr = new int[5]; // { 0, 0, 0, 0, 0 }
int[] nums = { 1, 3, 2, 5, 4 };

cpp：

/* 初始化数组 */
// 存储在栈上
int arr[5];
int nums[5] = { 1, 3, 2, 5, 4 };
// 存储在堆上（需要手动释放空间）
int* arr1 = new int[5];
int* nums1 = new int[5] { 1, 3, 2, 5, 4 };

访问元素

索引本质上是内存地址的偏移量。

在数组中访问元素非常高效，我们可以在?O(1)?时间内随机访问数组中的任意一个元素。

/* 随机访问元素 */
int randomAccess(int[] nums) {
    // 在区间 [0, nums.length) 中随机抽取一个数字
    int randomIndex = ThreadLocalRandom.current().nextInt(0, nums.length);
    // 获取并返回随机元素
    int randomNum = nums[randomIndex];
    return randomNum;
}
/* 随机访问元素 */
int randomAccess(int *nums, int size) {
    // 在区间 [0, size) 中随机抽取一个数字
    int randomIndex = rand() % size;
    // 获取并返回随机元素
    int randomNum = nums[randomIndex];
    return randomNum;
}

这里Java和cpp中用于在一定范围内随机抽取一个数字用的方法是不一样。

插入元素

数组元素在内存中是“紧挨着的”，它们之间没有空间再存放任何数据。如图 所示，如果想在数组中间插入一个元素，则需要将该元素之后的所有元素都向后移动一位，之后再把元素赋值给该索引。

?

/* 在数组的索引 index 处插入元素 num */
void insert(int[] nums, int num, int index) {
    // 把索引 index 以及之后的所有元素向后移动一位
    for (int i = nums.length - 1; i > index; i--) {
        nums[i] = nums[i - 1];
    }
    // 将 num 赋给 index 处的元素
    nums[index] = num;
}
/* 在数组的索引 index 处插入元素 num */
void insert(int *nums, int size, int num, int index) {
    // 把索引 index 以及之后的所有元素向后移动一位
    for (int i = size - 1; i > index; i--) {
        nums[i] = nums[i - 1];
    }
    // 将 num 赋给 index 处的元素
    nums[index] = num;
}

删除元素?

?同理，如图 4-4 所示，若想删除索引?i处的元素，则需要把索引i?之后的元素都向前移动一位。

/* 删除索引 index 处的元素 */
void remove(int[] nums, int index) {
    // 把索引 index 之后的所有元素向前移动一位
    for (int i = index; i < nums.length - 1; i++) {
        nums[i] = nums[i + 1];
    }
}
/* 删除索引 index 处的元素 */
void remove(int *nums, int size, int index) {
    // 把索引 index 之后的所有元素向前移动一位
    for (int i = index; i < size - 1; i++) {
        nums[i] = nums[i + 1];
    }
}

总的来看，数组的插入与删除操作有以下缺点。

  • 时间复杂度高：数组的插入和删除的平均时间复杂度均为O(n)，其中n为数组长度。
  • 丢失元素：由于数组的长度不可变，因此在插入元素后，超出数组长度范围的元素会丢失。
  • 内存浪费：我们可以初始化一个比较长的数组，只用前面一部分，这样在插入数据时，丢失的末尾元素都是“无意义”的，但这样做会造成部分内存空间浪费。

遍历数组：

/* 遍历数组 */
void traverse(int[] nums) {
    int count = 0;
    // 通过索引遍历数组
    for (int i = 0; i < nums.length; i++) {
        count += nums[i];
    }
    // 直接遍历数组元素
    for (int num : nums) {
        count += num;
    }
}
/* 遍历数组 */
void traverse(int *nums, int size) {
    int count = 0;
    // 通过索引遍历数组
    for (int i = 0; i < size; i++) {
        count += nums[i];
    }
}

查找元素?

在数组中查找指定元素需要遍历数组，每轮判断元素值是否匹配，若匹配则输出对应索引。

因为数组是线性数据结构，所以上述查找操作被称为“线性查找”。

/* 在数组中查找指定元素 */
int find(int[] nums, int target) {
    for (int i = 0; i < nums.length; i++) {
        if (nums[i] == target)
            return i;
    }
    return -1;
}
/* 在数组中查找指定元素 */
int find(int *nums, int size, int target) {
    for (int i = 0; i < size; i++) {
        if (nums[i] == target)
            return i;
    }
    return -1;
}

扩容数组：

在复杂的系统环境中，程序难以保证数组之后的内存空间是可用的，从而无法安全地扩展数组容量。因此在大多数编程语言中，数组的长度是不可变的。

如果我们希望扩容数组，则需重新建立一个更大的数组，然后把原数组元素依次复制到新数组。这是一个?O（n）的操作，在数组很大的情况下非常耗时。代码如下所示：

/* 扩展数组长度 */
int[] extend(int[] nums, int enlarge) {
    // 初始化一个扩展长度后的数组
    int[] res = new int[nums.length + enlarge];
    // 将原数组中的所有元素复制到新数组
    for (int i = 0; i < nums.length; i++) {
        res[i] = nums[i];
    }
    // 返回扩展后的新数组
    return res;
}
/* 扩展数组长度 */
int *extend(int *nums, int size, int enlarge) {
    // 初始化一个扩展长度后的数组
    int *res = new int[size + enlarge];
    // 将原数组中的所有元素复制到新数组
    for (int i = 0; i < size; i++) {
        res[i] = nums[i];
    }
    // 释放内存
    delete[] nums;
    // 返回扩展后的新数组
    return res;
}

数组的优点与局限性

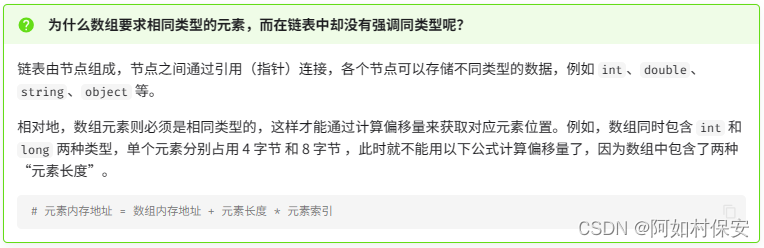
数组存储在连续的内存空间内，且元素类型相同。这种做法包含丰富的先验信息，系统可以利用这些信息来优化数据结构的操作效率。

  • 空间效率高：数组为数据分配了连续的内存块，无须额外的结构开销。
  • 支持随机访问：数组允许在?O(1)?时间内访问任何元素。
  • 缓存局部性：当访问数组元素时，计算机不仅会加载它，还会缓存其周围的其他数据，从而借助高速缓存来提升后续操作的执行速度。

连续空间存储是一把双刃剑，其存在以下局限性。

  • 插入与删除效率低：当数组中元素较多时，插入与删除操作需要移动大量的元素。
  • 长度不可变：数组在初始化后长度就固定了，扩容数组需要将所有数据复制到新数组，开销很大。
  • 空间浪费：如果数组分配的大小超过实际所需，那么多余的空间就被浪费了。

2 链表

「链表 linked list」是一种线性数据结构，其中的每个元素都是一个节点对象，各个节点通过“引用”相连接。引用记录了下一个节点的内存地址，通过它可以从当前节点访问到下一个节点。

链表的设计使得各个节点可以分散存储在内存各处，它们的内存地址无须连续。

?

链表的组成单位是「节点 node」对象。每个节点都包含两项数据：节点的“值”和指向下一节点的“引用”。

  • 链表的首个节点被称为“头节点”，最后一个节点被称为“尾节点”。
  • 尾节点指向的是“空”，它在 Java、C++ 和 Python 中分别被记为?null、nullptr?和?None?。
  • 在 C、C++、Go 和 Rust 等支持指针的语言中，上述“引用”应被替换为“指针”。

如以下代码所示，链表节点?ListNode?除了包含值，还需额外保存一个引用（指针）。因此在相同数据量下，链表比数组占用更多的内存空间。

/* 链表节点类 */
class ListNode {
    int val;        // 节点值
    ListNode next;  // 指向下一节点的引用
    ListNode(int x) { val = x; }  // 构造函数
}
/* 链表节点结构体 */
struct ListNode {
    int val;         // 节点值
    ListNode *next;  // 指向下一节点的指针
    ListNode(int x) : val(x), next(nullptr) {}  // 构造函数
};

初始化链表

建立链表分为两步，第一步是初始化各个节点对象，第二步是构建节点之间的引用关系。初始化完成后，我们就可以从链表的头节点出发，通过引用指向?next?依次访问所有节点。

/* 初始化链表 1 -> 3 -> 2 -> 5 -> 4 */
// 初始化各个节点
ListNode n0 = new ListNode(1);
ListNode n1 = new ListNode(3);
ListNode n2 = new ListNode(2);
ListNode n3 = new ListNode(5);
ListNode n4 = new ListNode(4);
// 构建节点之间的引用
n0.next = n1;
n1.next = n2;
n2.next = n3;
n3.next = n4;
/* 初始化链表 1 -> 3 -> 2 -> 5 -> 4 */
// 初始化各个节点
ListNode* n0 = new ListNode(1);
ListNode* n1 = new ListNode(3);
ListNode* n2 = new ListNode(2);
ListNode* n3 = new ListNode(5);
ListNode* n4 = new ListNode(4);
// 构建节点之间的引用
n0->next = n1;
n1->next = n2;
n2->next = n3;
n3->next = n4;

数组整体是一个变量，比如数组?nums?包含元素?nums[0]?和?nums[1]?等，而链表是由多个独立的节点对象组成的。我们通常将头节点当作链表的代称，比如以上代码中的链表可记作链表?n0?。

插入节点

在链表中插入节点非常容易。如图 4-6 所示，假设我们想在相邻的两个节点?n0?和?n1?之间插入一个新节点?P?，则只需改变两个节点引用（指针）即可，时间复杂度为?O(1)?。

相比之下，在数组中插入元素的时间复杂度为?O(n)?，在大数据量下的效率较低。

?

/* 在链表的节点 n0 之后插入节点 P */
void insert(ListNode n0, ListNode P) {
    ListNode n1 = n0.next;
    P.next = n1;
    n0.next = P;
}
/* 在链表的节点 n0 之后插入节点 P */
void insert(ListNode *n0, ListNode *P) {
    ListNode *n1 = n0->next;
    P->next = n1;
    n0->next = P;
}

删除节点

在链表中删除节点也非常方便，只需改变一个节点的引用（指针）即可。

请注意，尽管在删除操作完成后节点?P?仍然指向?n1?，但实际上遍历此链表已经无法访问到?P?，这意味着?P?已经不再属于该链表了。

?

/* 删除链表的节点 n0 之后的首个节点 */
void remove(ListNode n0) {
    if (n0.next == null)
        return;
    // n0 -> P -> n1
    ListNode P = n0.next;
    ListNode n1 = P.next;
    n0.next = n1;
}
/* 删除链表的节点 n0 之后的首个节点 */
void remove(ListNode *n0) {
    if (n0->next == nullptr)
        return;
    // n0 -> P -> n1
    ListNode *P = n0->next;
    ListNode *n1 = P->next;
    n0->next = n1;
    // 释放内存
    delete P;
}

访问节点

在链表中访问节点的效率较低。如上一节所述，我们可以在?O(1)?时间下访问数组中的任意元素。链表则不然，程序需要从头节点出发，逐个向后遍历，直至找到目标节点。也就是说，访问链表的第 i?个节点需要循环?i?1?轮，时间复杂度为O(n)?。

/* 访问链表中索引为 index 的节点 */
ListNode access(ListNode head, int index) {
    for (int i = 0; i < index; i++) {
        if (head == null)
            return null;
        head = head.next;
    }
    return head;
}
/* 访问链表中索引为 index 的节点 */
ListNode *access(ListNode *head, int index) {
    for (int i = 0; i < index; i++) {
        if (head == nullptr)
            return nullptr;
        head = head->next;
    }
    return head;
}

查找节点

遍历链表，查找其中值为?target?的节点，输出该节点在链表中的索引。此过程也属于线性查找。代码如下所示：

/* 在链表中查找值为 target 的首个节点 */
int find(ListNode head, int target) {
    int index = 0;
    while (head != null) {
        if (head.val == target)
            return index;
        head = head.next;
        index++;
    }
    return -1;
}
/* 在链表中查找值为 target 的首个节点 */
int find(ListNode *head, int target) {
    int index = 0;
    while (head != nullptr) {
        if (head->val == target)
            return index;
        head = head->next;
        index++;
    }
    return -1;
}

数组 vs. 链表

?

常见链表类型

如图所示，常见的链表类型包括三种。

  • 单向链表：即前面介绍的普通链表。单向链表的节点包含值和指向下一节点的引用两项数据。我们将首个节点称为头节点，将最后一个节点称为尾节点，尾节点指向空?None?。
  • 环形链表：如果我们令单向链表的尾节点指向头节点（首尾相接），则得到一个环形链表。在环形链表中，任意节点都可以视作头节点。
  • 双向链表：与单向链表相比，双向链表记录了两个方向的引用。双向链表的节点定义同时包含指向后继节点（下一个节点）和前驱节点（上一个节点）的引用（指针）。相较于单向链表，双向链表更具灵活性，可以朝两个方向遍历链表，但相应地也需要占用更多的内存空间。
/* 双向链表节点类 */
class ListNode {
    int val;        // 节点值
    ListNode next;  // 指向后继节点的引用
    ListNode prev;  // 指向前驱节点的引用
    ListNode(int x) { val = x; }  // 构造函数
}
/* 双向链表节点结构体 */
struct ListNode {
    int val;         // 节点值
    ListNode *next;  // 指向后继节点的指针
    ListNode *prev;  // 指向前驱节点的指针
    ListNode(int x) : val(x), next(nullptr), prev(nullptr) {}  // 构造函数
};

?

3?列表

「列表 list」是一个抽象的数据结构概念，它表示元素的有序集合，支持元素访问、修改、添加、删除和遍历等操作，无须使用者考虑容量限制的问题。列表可以基于链表或数组实现。

  • 链表天然可以看作一个列表，其支持元素增删查改操作，并且可以灵活动态扩容。
  • 数组也支持元素增删查改，但由于其长度不可变，因此只能看作一个具有长度限制的列表。

当使用数组实现列表时，长度不可变的性质会导致列表的实用性降低。这是因为我们通常无法事先确定需要存储多少数据，从而难以选择合适的列表长度。若长度过小，则很可能无法满足使用需求；若长度过大，则会造成内存空间浪费。

为解决此问题，我们可以使用「动态数组 dynamic array」来实现列表。它继承了数组的各项优点，并且可以在程序运行过程中进行动态扩容。

实际上，许多编程语言中的标准库提供的列表是基于动态数组实现的，例如 Python 中的?list?、Java 中的?ArrayList?、C++ 中的?vector?和 C# 中的?List?等。在接下来的讨论中，我们将把“列表”和“动态数组”视为等同的概念。

初始化列表

/* 初始化列表 */
// 无初始值
List<Integer> nums1 = new ArrayList<>();
// 有初始值（注意数组的元素类型需为 int[] 的包装类 Integer[]）
Integer[] numbers = new Integer[] { 1, 3, 2, 5, 4 };
List<Integer> nums = new ArrayList<>(Arrays.asList(numbers));
/* 初始化列表 */
// 需注意，C++ 中 vector 即是本文描述的 nums
// 无初始值
vector<int> nums1;
// 有初始值
vector<int> nums = { 1, 3, 2, 5, 4 };

访问元素

列表本质上是数组，因此可以在?O(1)?时间内访问和更新元素，效率很高。

/* 访问元素 */
int num = nums.get(1);  // 访问索引 1 处的元素

/* 更新元素 */
nums.set(1, 0);  // 将索引 1 处的元素更新为 0
/* 访问元素 */
int num = nums[1];  // 访问索引 1 处的元素

/* 更新元素 */
nums[1] = 0;  // 将索引 1 处的元素更新为 0

插入与删除元素

相较于数组，列表可以自由地添加与删除元素。在列表尾部添加元素的时间复杂度为?O(1)?，但插入和删除元素的效率仍与数组相同，时间复杂度为?O(n)?。

/* 清空列表 */
nums.clear();

/* 在尾部添加元素 */
nums.add(1);
nums.add(3);
nums.add(2);
nums.add(5);
nums.add(4);

/* 在中间插入元素 */
nums.add(3, 6);  // 在索引 3 处插入数字 6

/* 删除元素 */
nums.remove(3);  // 删除索引 3 处的元素
/* 清空列表 */
nums.clear();

/* 在尾部添加元素 */
nums.push_back(1);
nums.push_back(3);
nums.push_back(2);
nums.push_back(5);
nums.push_back(4);

/* 在中间插入元素 */
nums.insert(nums.begin() + 3, 6);  // 在索引 3 处插入数字 6

/* 删除元素 */
nums.erase(nums.begin() + 3);      // 删除索引 3 处的元素

遍历列表

与数组一样，列表可以根据索引遍历，也可以直接遍历各元素。

/* 通过索引遍历列表 */
int count = 0;
for (int i = 0; i < nums.size(); i++) {
    count += nums.get(i);
}

/* 直接遍历列表元素 */
for (int num : nums) {
    count += num;
}
/* 通过索引遍历列表 */
int count = 0;
for (int i = 0; i < nums.size(); i++) {
    count += nums[i];
}

/* 直接遍历列表元素 */
count = 0;
for (int num : nums) {
    count += num;
}

拼接列表

给定一个新列表?nums1?，我们可以将其拼接到原列表的尾部。

/* 拼接两个列表 */
List<Integer> nums1 = new ArrayList<>(Arrays.asList(new Integer[] { 6, 8, 7, 10, 9 }));
nums.addAll(nums1);  // 将列表 nums1 拼接到 nums 之后
/* 拼接两个列表 */
vector<int> nums1 = { 6, 8, 7, 10, 9 };
// 将列表 nums1 拼接到 nums 之后
nums.insert(nums.end(), nums1.begin(), nums1.end());

排序列表

完成列表排序后，我们便可以使用在数组类算法题中经常考查的“二分查找”和“双指针”算法。

/* 排序列表 */
Collections.sort(nums);  // 排序后，列表元素从小到大排列
/* 排序列表 */
sort(nums.begin(), nums.end());  // 排序后，列表元素从小到大排列

列表实现

简易版列表，包括以下三个重点设计。

  • 初始容量：选取一个合理的数组初始容量。在本示例中，我们选择 10 作为初始容量。
  • 数量记录：声明一个变量?size?，用于记录列表当前元素数量，并随着元素插入和删除实时更新。根据此变量，我们可以定位列表尾部，以及判断是否需要扩容。
  • 扩容机制：若插入元素时列表容量已满，则需要进行扩容。先根据扩容倍数创建一个更大的数组，再将当前数组的所有元素依次移动至新数组。在本示例中，我们规定每次将数组扩容至之前的 2 倍。
/* 列表类 */
class MyList {
    private int[] arr; // 数组（存储列表元素）
    private int capacity = 10; // 列表容量
    private int size = 0; // 列表长度（当前元素数量）
    private int extendRatio = 2; // 每次列表扩容的倍数

    /* 构造方法 */
    public MyList() {
        arr = new int[capacity];
    }

    /* 获取列表长度（当前元素数量） */
    public int size() {
        return size;
    }

    /* 获取列表容量 */
    public int capacity() {
        return capacity;
    }

    /* 访问元素 */
    public int get(int index) {
        // 索引如果越界，则抛出异常，下同
        if (index < 0 || index >= size)
            throw new IndexOutOfBoundsException("索引越界");
        return arr[index];
    }

    /* 更新元素 */
    public void set(int index, int num) {
        if (index < 0 || index >= size)
            throw new IndexOutOfBoundsException("索引越界");
        arr[index] = num;
    }

    /* 在尾部添加元素 */
    public void add(int num) {
        // 元素数量超出容量时，触发扩容机制
        if (size == capacity())
            extendCapacity();
        arr[size] = num;
        // 更新元素数量
        size++;
    }

    /* 在中间插入元素 */
    public void insert(int index, int num) {
        if (index < 0 || index >= size)
            throw new IndexOutOfBoundsException("索引越界");
        // 元素数量超出容量时，触发扩容机制
        if (size == capacity())
            extendCapacity();
        // 将索引 index 以及之后的元素都向后移动一位
        for (int j = size - 1; j >= index; j--) {
            arr[j + 1] = arr[j];
        }
        arr[index] = num;
        // 更新元素数量
        size++;
    }

    /* 删除元素 */
    public int remove(int index) {
        if (index < 0 || index >= size)
            throw new IndexOutOfBoundsException("索引越界");
        int num = arr[index];
        // 将索引 index 之后的元素都向前移动一位
        for (int j = index; j < size - 1; j++) {
            arr[j] = arr[j + 1];
        }
        // 更新元素数量
        size--;
        // 返回被删除的元素
        return num;
    }

    /* 列表扩容 */
    public void extendCapacity() {
        // 新建一个长度为原数组 extendRatio 倍的新数组，并将原数组复制到新数组
        arr = Arrays.copyOf(arr, capacity() * extendRatio);
        // 更新列表容量
        capacity = arr.length;
    }

    /* 将列表转换为数组 */
    public int[] toArray() {
        int size = size();
        // 仅转换有效长度范围内的列表元素
        int[] arr = new int[size];
        for (int i = 0; i < size; i++) {
            arr[i] = get(i);
        }
        return arr;
    }
}
/* 列表类 */
class MyList {
  private:
    int *arr;             // 数组（存储列表元素）
    int arrCapacity = 10; // 列表容量
    int arrSize = 0;      // 列表长度（当前元素数量）
    int extendRatio = 2;   // 每次列表扩容的倍数

  public:
    /* 构造方法 */
    MyList() {
        arr = new int[arrCapacity];
    }

    /* 析构方法 */
    ~MyList() {
        delete[] arr;
    }

    /* 获取列表长度（当前元素数量）*/
    int size() {
        return arrSize;
    }

    /* 获取列表容量 */
    int capacity() {
        return arrCapacity;
    }

    /* 访问元素 */
    int get(int index) {
        // 索引如果越界，则抛出异常，下同
        if (index < 0 || index >= size())
            throw out_of_range("索引越界");
        return arr[index];
    }

    /* 更新元素 */
    void set(int index, int num) {
        if (index < 0 || index >= size())
            throw out_of_range("索引越界");
        arr[index] = num;
    }

    /* 在尾部添加元素 */
    void add(int num) {
        // 元素数量超出容量时，触发扩容机制
        if (size() == capacity())
            extendCapacity();
        arr[size()] = num;
        // 更新元素数量
        arrSize++;
    }

    /* 在中间插入元素 */
    void insert(int index, int num) {
        if (index < 0 || index >= size())
            throw out_of_range("索引越界");
        // 元素数量超出容量时，触发扩容机制
        if (size() == capacity())
            extendCapacity();
        // 将索引 index 以及之后的元素都向后移动一位
        for (int j = size() - 1; j >= index; j--) {
            arr[j + 1] = arr[j];
        }
        arr[index] = num;
        // 更新元素数量
        arrSize++;
    }

    /* 删除元素 */
    int remove(int index) {
        if (index < 0 || index >= size())
            throw out_of_range("索引越界");
        int num = arr[index];
        // 索引 i 之后的元素都向前移动一位
        for (int j = index; j < size() - 1; j++) {
            arr[j] = arr[j + 1];
        }
        // 更新元素数量
        arrSize--;
        // 返回被删除的元素
        return num;
    }

    /* 列表扩容 */
    void extendCapacity() {
        // 新建一个长度为原数组 extendRatio 倍的新数组
        int newCapacity = capacity() * extendRatio;
        int *tmp = arr;
        arr = new int[newCapacity];
        // 将原数组中的所有元素复制到新数组
        for (int i = 0; i < size(); i++) {
            arr[i] = tmp[i];
        }
        // 释放内存
        delete[] tmp;
        arrCapacity = newCapacity;
    }

    /* 将列表转换为 Vector 用于打印 */
    vector<int> toVector() {
        // 仅转换有效长度范围内的列表元素
        vector<int> vec(size());
        for (int i = 0; i < size(); i++) {
            vec[i] = arr[i];
        }
        return vec;
    }
};

4?内存与缓存

物理结构在很大程度上决定了程序对内存和缓存的使用效率，进而影响算法程序的整体性能。

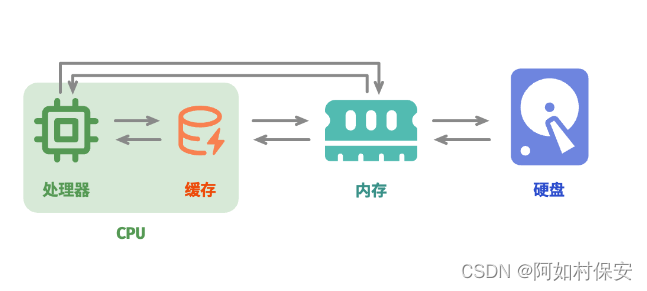
计算机中包括三种类型的存储设备：「硬盘 hard disk」、「内存 random-access memory, RAM」、「缓存 cache memory」。表展示了它们在计算机系统中的不同角色和性能特点。

?

越靠近金字塔顶端的存储设备的速度越快、容量越小、成本越高。这种多层级的设计并非偶然，而是计算机科学家和工程师们经过深思熟虑的结果。

  • 硬盘难以被内存取代。首先，内存中的数据在断电后会丢失，因此它不适合长期存储数据；其次，内存的成本是硬盘的几十倍，这使得它难以在消费者市场普及。
  • 缓存的大容量和高速度难以兼得。随着 L1、L2、L3 缓存的容量逐步增大，其物理尺寸会变大，与 CPU 核心之间的物理距离会变远，从而导致数据传输时间增加，元素访问延迟变高。在当前技术下，多层级的缓存结构是容量、速度和成本之间的最佳平衡点。

?

计算机的存储层次结构体现了速度、容量和成本三者之间的精妙平衡。实际上，这种权衡普遍存在于所有工业领域，它要求我们在不同的优势和限制之间找到最佳平衡点。

总的来说，硬盘用于长期存储大量数据，内存用于临时存储程序运行中正在处理的数据，而缓存则用于存储经常访问的数据和指令，以提高程序运行效率。三者共同协作，确保计算机系统高效运行。

如图所示，在程序运行时，数据会从硬盘中被读取到内存中，供 CPU 计算使用。缓存可以看作 CPU 的一部分，它通过智能地从内存加载数据，给 CPU 提供高速的数据读取，从而显著提升程序的执行效率，减少对较慢的内存的依赖。

?

总结

?

?

?

?

?

?

?

语言真的烦的很，不同的项目就要用不同的语言，所以都复习一下，但是很容易搞混。

这里的复习参考是：

第 4 章 ? 数组与链表 - Hello 算法 (hello-algo.com)

文章来源:https://blog.csdn.net/m0_66076989/article/details/135393310
本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。
最新文章