Leetcode刷题笔记——快速幂算法之万物皆可分治
发布时间:2023年12月18日
递归法
算法思想

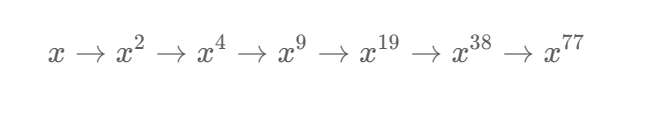

代码实现
1. 清晰易懂版
class Solution {
public:
double myPow(double x, long int n) {
if (n == 0) return 1;
if (n < 0){
return 1 / myPow(x,-n);
}
if (n % 2){
return x * myPow(x,n-1);
}
return myPow(x*x,n/2);
}
};2. 拆解成两个函数版?
class Solution {
public:
double quickMul(double x, long long N) {
if (N == 0) {
return 1.0;
}
double y = quickMul(x, N / 2);
return N % 2 == 0 ? y * y : y * y * x;
}
double myPow(double x, int n) {
long long N = n;
return N >= 0 ? quickMul(x, N) : 1.0 / quickMul(x, -N);
}
};
时空复杂度:
时间复杂度:O(log?n)
空间复杂度:O(log?n),即为递归的层数
迭代法
算法思想


代码实现
1. 一个函数版
class Solution {
// 迭代算法,利用二进制位
public double myPow(double x, int n) {
if(x == 0) return x;
long power = n; // 为了保证-n不溢出,先转换成long类型
if(n < 0){ // 如果n小于0, 求1/x的-n次方
power *= -1;
x = 1 / x;
}
double weight = x; // 权值初值为x, 即二进制位第1位的权值为x^1
double res = 1;
while(power != 0){
// 如果当前二进制位为1, 让结果乘上这个二进制位上的权值,
// 该位权值在上一轮迭代中已经计算出来了
if((power & 1) == 1) res *= weight;
weight *= weight; // 计算下一个二进制位的权值
power >> 2;
}
return res;
}
}2. 两个函数版
class Solution {
public:
double qpow(double a, long long b){
double res = 1;
while(b){
if(b&1) res = res*a;
b >>= 1;
a *= a;
}
return res;
}
double myPow(double x, long long n) {
if(n == 0) return 1;
if(n > 0) return qpow(x,n);
if(n < 0) return 1/qpow(x,-n);
return 1.0;
}
};相关题目
文章来源:https://blog.csdn.net/weixin_53432918/article/details/134914751
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:chenni525@qq.com进行投诉反馈,一经查实,立即删除!
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:chenni525@qq.com进行投诉反馈,一经查实,立即删除!
最新文章
- Python教程
- 深入理解 MySQL 中的 HAVING 关键字和聚合函数
- Qt之QChar编码(1)
- MyBatis入门基础篇
- 用Python脚本实现FFmpeg批量转换
- JAVA电商平台 免 费 搭 建 B2B2C商城系统 多用户商城系统 直播带货 新零售商城 o2o商城 电子商务 拼团商城 分销商城
- doNet Core中解压zip
- gem5学习(8):创建一个简单的缓存对象--Creating a simple cache object
- 自己动手写数据库系统:解释执行 update 和 delete 对应的 sql 语句
- 基于D2-NET的图像配准(基于PYQT的可运行界面)
- 【教学类-43-11】 20231231 3*3宫格数独提取单元格坐标数字的通用模板(做成2*2=4套、3*2=6套)
- Oracle触发器简单应用示例
- 服装加工ERP软件包含哪些模块?可以帮助企业处理什么难题
- 美国智库发布《用人工智能展望网络未来》的解析
- Ubuntu设置kubelet启动脚本关闭swap分区