python:五种算法(PSO、RFO、HHO、WOA、GWO)求解23个测试函数(python代码)
发布时间:2023年12月17日
一、五种算法简介
1、粒子群优化算法PSO
2、红狐优化算法RFO
3、哈里斯鹰优化算法HHO
4、鲸鱼优化算法WOA
5、灰狼优化算法GWO
二、5种算法求解23个函数
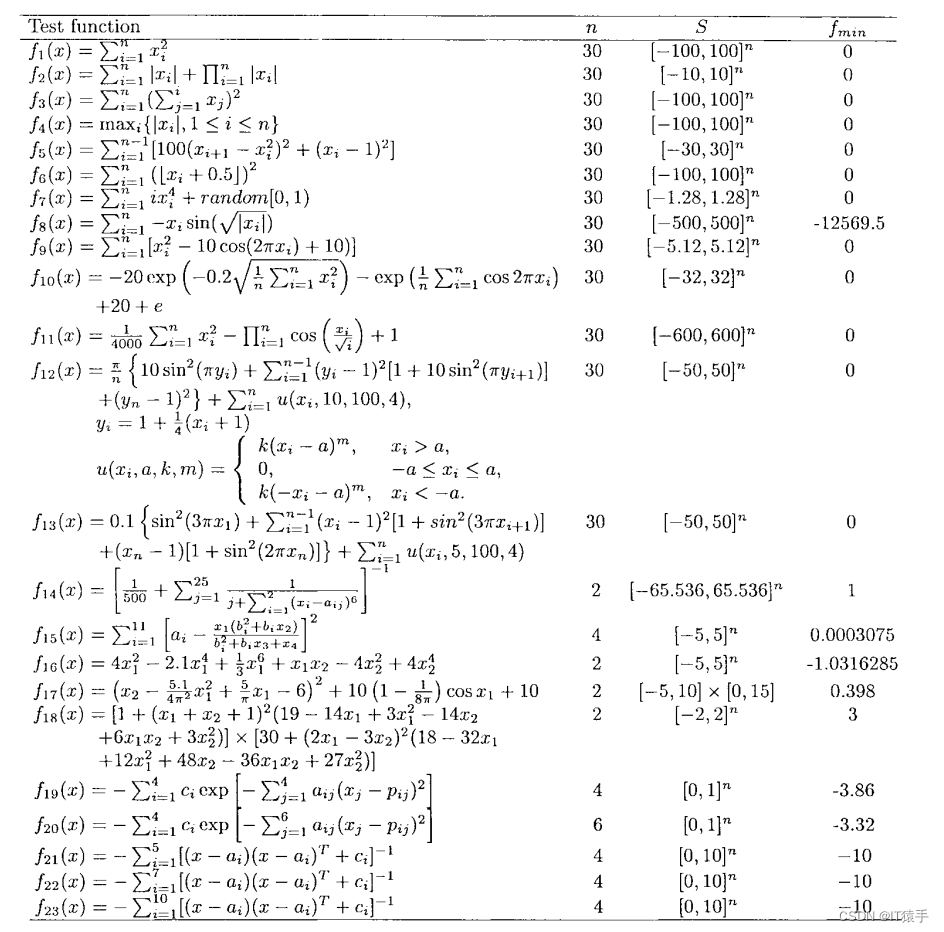
(1)23个函数简介
参考文献:
[1] Yao X, Liu Y, Lin G M. Evolutionary programming made faster[J]. IEEE transactions on evolutionary?
(2)部分python代码
from FunInfo import Get_Functions_details
from WOA import WOA
from GWO import GWO
from PSO import PSO
from RFO import RFO
from HHO import HHO
import matplotlib.pyplot as plt
from func_plot import func_plot
plt.rcParams['font.sans-serif']=['Microsoft YaHei']
#主程序
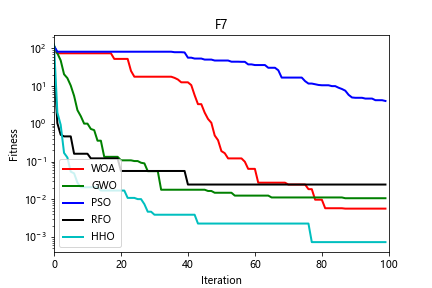
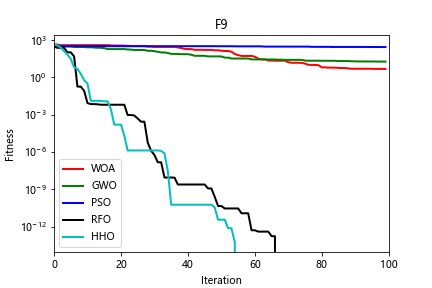
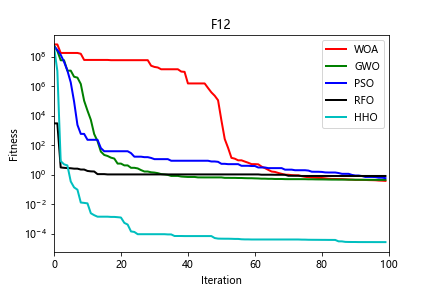
function_name =7 #测试函数1-23
SearchAgents_no = 50#种群大小
Max_iter = 100#迭代次数
lb,ub,dim,fobj=Get_Functions_details(function_name)#获取问题信息
BestX1,BestF1,curve1 = WOA(SearchAgents_no, Max_iter,lb,ub,dim,fobj)#问题求解
BestX2,BestF2,curve2 = GWO(SearchAgents_no, Max_iter,lb,ub,dim,fobj)#问题求解
BestX3,BestF3,curve3 = PSO(SearchAgents_no, Max_iter,lb,ub,dim,fobj)#问题求解
BestX4,BestF4,curve4 = RFO(SearchAgents_no, Max_iter,lb,ub,dim,fobj)#问题求解
BestX5,BestF5,curve5 = HHO(SearchAgents_no, Max_iter,lb,ub,dim,fobj)#问题求解
#画函数图
func_plot(lb,ub,dim,fobj,function_name)#画函数图
#画收敛曲线图
Labelstr=['WOA','GWO','PSO','RFO','HHO']
Colorstr=['r','g','b','k','c']
if BestF1>0:
? ? plt.semilogy(curve1,color=Colorstr[0],linewidth=2,label=Labelstr[0])
? ? plt.semilogy(curve2,color=Colorstr[1],linewidth=2,label=Labelstr[1])
? ? plt.semilogy(curve3,color=Colorstr[2],linewidth=2,label=Labelstr[2])
? ? plt.semilogy(curve4,color=Colorstr[3],linewidth=2,label=Labelstr[3])
? ? plt.semilogy(curve5,color=Colorstr[4],linewidth=2,label=Labelstr[4])
else:
? ? plt.plot(curve1,color=Colorstr[0],linewidth=2,label=Labelstr[0])
? ? plt.plot(curve2,color=Colorstr[1],linewidth=2,label=Labelstr[1])
? ? plt.plot(curve3,color=Colorstr[2],linewidth=2,label=Labelstr[2])
? ? plt.plot(curve4,color=Colorstr[3],linewidth=2,label=Labelstr[3])
? ? plt.plot(curve5,color=Colorstr[4],linewidth=2,label=Labelstr[4])
plt.xlabel("Iteration")
plt.ylabel("Fitness")
plt.xlim(0,Max_iter)
plt.title("F"+str(function_name))
plt.legend()
plt.savefig(str(function_name)+'.png')
plt.show()
#
(3)部分结果



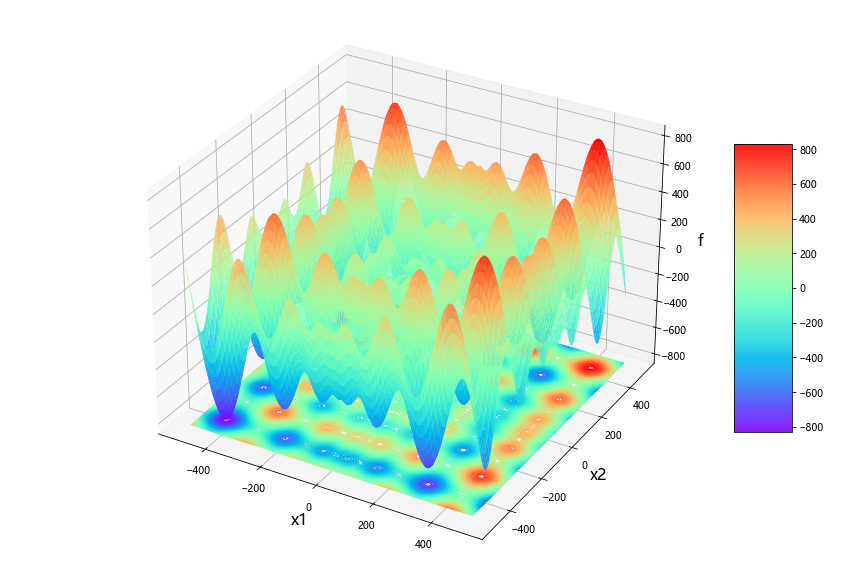

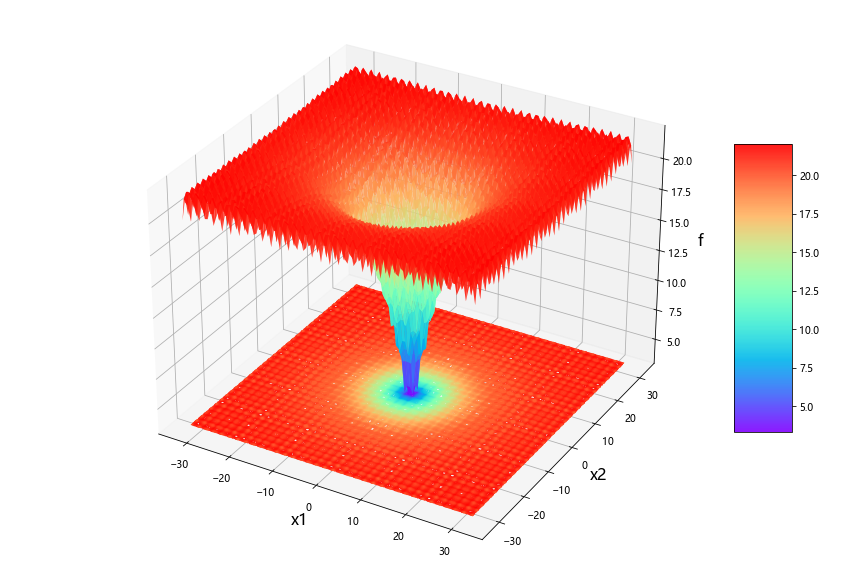
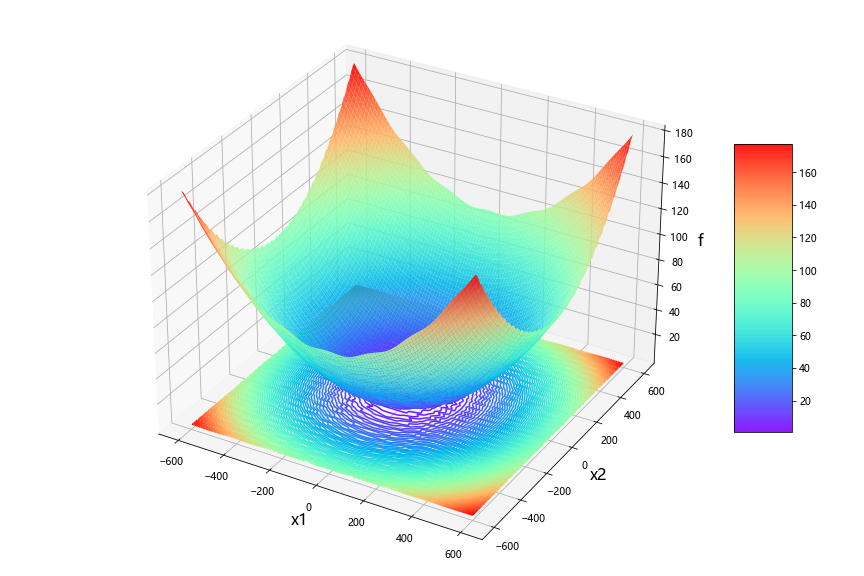

三、完整python代码
python:五种算法(PSO、RFO、HHO、WOA、GWO)求解23个测试函数(python代码) - 哔哩哔哩 (bilibili.com)
文章来源:https://blog.csdn.net/weixin_46204734/article/details/134980787
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:chenni525@qq.com进行投诉反馈,一经查实,立即删除!
本文来自互联网用户投稿,该文观点仅代表作者本人,不代表本站立场。本站仅提供信息存储空间服务,不拥有所有权,不承担相关法律责任。 如若内容造成侵权/违法违规/事实不符,请联系我的编程经验分享网邮箱:chenni525@qq.com进行投诉反馈,一经查实,立即删除!
最新文章
- Python教程
- 深入理解 MySQL 中的 HAVING 关键字和聚合函数
- Qt之QChar编码(1)
- MyBatis入门基础篇
- 用Python脚本实现FFmpeg批量转换
- HubSpot电子邮件:数字化时代的营销利器
- #初入versionMaster
- 主从复制的原理、方法及详解
- AI嵌入式K210项目(14)-TF卡读取
- C#入门篇(一)
- Leetcode—54.螺旋矩阵【中等】
- Nacos和Eureka的全面对比
- kafka消费Clock skew too great (37),CPU打爆
- 每日一练:LeeCode-503. 下一个更大元素 II (中)【单调栈】
- 数据结构 数组与字符串